The PHREG Procedure
- Overview
-
Getting Started
-
SyntaxPROC PHREG StatementASSESS StatementBASELINE StatementBAYES StatementBY StatementCLASS StatementCONTRAST StatementEFFECT StatementESTIMATE StatementFREQ StatementHAZARDRATIO StatementID StatementLSMEANS StatementLSMESTIMATE StatementMODEL StatementOUTPUT StatementProgramming StatementsRANDOM StatementSTRATA StatementSLICE StatementSTORE StatementTEST StatementWEIGHT Statement
-
DetailsFailure Time DistributionTime and CLASS Variables UsagePartial Likelihood Function for the Cox ModelCounting Process Style of InputLeft-Truncation of Failure TimesThe Multiplicative Hazards ModelProportional Rates/Means Models for Recurrent EventsThe Frailty ModelProportional Subdistribution Hazards Model for Competing-Risks DataHazard RatiosNewton-Raphson MethodFirth’s Modification for Maximum Likelihood EstimationRobust Sandwich Variance EstimateTesting the Global Null HypothesisType 3 Tests and Joint TestsConfidence Limits for a Hazard RatioUsing the TEST Statement to Test Linear HypothesesAnalysis of Multivariate Failure Time DataModel Fit StatisticsSchemper-Henderson Predictive MeasureResidualsDiagnostics Based on Weighted ResidualsInfluence of Observations on Overall Fit of the ModelSurvivor Function EstimatorsCaution about Using Survival Data with Left TruncationEffect Selection MethodsAssessment of the Proportional Hazards ModelThe Penalized Partial Likelihood Approach for Fitting Frailty ModelsSpecifics for Bayesian AnalysisComputational ResourcesInput and Output Data SetsDisplayed OutputODS Table NamesODS Graphics
-
ExamplesStepwise RegressionBest Subset SelectionModeling with Categorical PredictorsFirth’s Correction for Monotone LikelihoodConditional Logistic Regression for m:n MatchingModel Using Time-Dependent Explanatory VariablesTime-Dependent Repeated Measurements of a CovariateSurvival CurvesAnalysis of ResidualsAnalysis of Recurrent Events DataAnalysis of Clustered DataModel Assessment Using Cumulative Sums of Martingale ResidualsBayesian Analysis of the Cox ModelBayesian Analysis of Piecewise Exponential ModelAnalysis of Competing-Risks Data
- References
Failure Time Distribution
Let T be a nonnegative random variable representing the failure time of an individual from a homogeneous population. The survival distribution function (also known as the survivor function) of T is written as
![\[ S(t)=\mr{Pr}(T \geq t) \]](images/statug_phreg0185.png)
A mathematically equivalent way of specifying the distribution of T is through its hazard function. The hazard function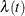 
specifies the instantaneous failure rate at t. If T is a continuous random variable, 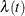is expressed as
![\[ \lambda (t)= \lim _{\Delta t \rightarrow 0^{+} } \frac{ \mr{Pr}(t \leq T < t + \Delta t\ |\ T \geq t) }{ \Delta t } = \frac{f(t)}{S(t)} \]](images/statug_phreg0187.png)
where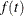 
is the probability density function of T. If T is discrete with masses at 
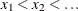, then survivor function is given by
![\[ S(t)= \sum _{x_ j\leq t} \mr{Pr}(T=x_ j) = \sum _{j} \mr{Pr}(T=j) \delta (t- x_{j}) \]](images/statug_phreg0190.png)
where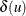 
=0 if u < 0 and 
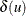=1 otherwise. The discrete hazards are given by
![\[ \lambda _{j}=\mr{Pr}(T=x_{j}\ |\ T \geq x_{j})=\frac{ \mr{Pr}(T=x_{j}) }{S(x_{j}) } \mbox{~ ~ ~ } j=1, 2, \ldots \]](images/statug_phreg0193.png)