The SEQDESIGN Procedure
-
Overview

- Getting Started
-
Syntax

-
Details
 Fixed-Sample Clinical TrialsOne-Sided Fixed-Sample Tests in Clinical TrialsTwo-Sided Fixed-Sample Tests in Clinical TrialsGroup Sequential MethodsStatistical Assumptions for Group Sequential DesignsBoundary ScalesBoundary VariablesType I and Type II ErrorsUnified Family MethodsHaybittle-Peto MethodWhitehead MethodsError Spending MethodsAcceptance (beta) BoundaryBoundary Adjustments for Overlapping Lower and Upper beta BoundariesSpecified and Derived ParametersApplicable Boundary KeysSample Size ComputationApplicable One-Sample Tests and Sample Size ComputationApplicable Two-Sample Tests and Sample Size ComputationApplicable Regression Parameter Tests and Sample Size ComputationAspects of Group Sequential DesignsSummary of Methods in Group Sequential DesignsTable OutputODS Table NamesGraphics OutputODS Graphics
Fixed-Sample Clinical TrialsOne-Sided Fixed-Sample Tests in Clinical TrialsTwo-Sided Fixed-Sample Tests in Clinical TrialsGroup Sequential MethodsStatistical Assumptions for Group Sequential DesignsBoundary ScalesBoundary VariablesType I and Type II ErrorsUnified Family MethodsHaybittle-Peto MethodWhitehead MethodsError Spending MethodsAcceptance (beta) BoundaryBoundary Adjustments for Overlapping Lower and Upper beta BoundariesSpecified and Derived ParametersApplicable Boundary KeysSample Size ComputationApplicable One-Sample Tests and Sample Size ComputationApplicable Two-Sample Tests and Sample Size ComputationApplicable Regression Parameter Tests and Sample Size ComputationAspects of Group Sequential DesignsSummary of Methods in Group Sequential DesignsTable OutputODS Table NamesGraphics OutputODS Graphics -
Examples
 Creating Fixed-Sample DesignsCreating a One-Sided O’Brien-Fleming DesignCreating Two-Sided Pocock and O’Brien-Fleming DesignsGenerating Graphics Display for Sequential DesignsCreating Designs Using Haybittle-Peto MethodsCreating Designs with Various Stopping CriteriaCreating Whitehead’s Triangular DesignsCreating a One-Sided Error Spending DesignCreating Designs with Various Number of StagesCreating Two-Sided Error Spending Designs with and without Overlapping Lower and Upper beta BoundariesCreating a Two-Sided Asymmetric Error Spending Design with Early Stopping to Reject H0Creating a Two-Sided Asymmetric Error Spending Design with Early Stopping to Reject or Accept H0Creating a Design with a Nonbinding Beta BoundaryComputing Sample Size for Survival Data That Have Uniform AccrualComputing Sample Size for Survival Data with Truncated Exponential Accrual
Creating Fixed-Sample DesignsCreating a One-Sided O’Brien-Fleming DesignCreating Two-Sided Pocock and O’Brien-Fleming DesignsGenerating Graphics Display for Sequential DesignsCreating Designs Using Haybittle-Peto MethodsCreating Designs with Various Stopping CriteriaCreating Whitehead’s Triangular DesignsCreating a One-Sided Error Spending DesignCreating Designs with Various Number of StagesCreating Two-Sided Error Spending Designs with and without Overlapping Lower and Upper beta BoundariesCreating a Two-Sided Asymmetric Error Spending Design with Early Stopping to Reject H0Creating a Two-Sided Asymmetric Error Spending Design with Early Stopping to Reject or Accept H0Creating a Design with a Nonbinding Beta BoundaryComputing Sample Size for Survival Data That Have Uniform AccrualComputing Sample Size for Survival Data with Truncated Exponential Accrual - References
Type I and Type II Errors
The Type I error is the error of rejecting the null hypothesis when the null hypothesis is correct, and the Type II error
is the error of not rejecting the null hypothesis when the null hypothesis is incorrect. The level of significance  is the probability of making a Type I error. The Type II error depends on the hypothetical reference of the alternative hypothesis,
and the Type II error probability
is the probability of making a Type I error. The Type II error depends on the hypothetical reference of the alternative hypothesis,
and the Type II error probability 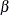 is defined as the probability of not rejecting the null hypothesis when a specific alternative reference is true. The power
is defined as the probability of not rejecting the null hypothesis when a specific alternative reference is true. The power
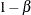 is then defined as the probability of rejecting the null hypothesis at the alternative reference.
is then defined as the probability of rejecting the null hypothesis at the alternative reference.
In a sequential design, if the maximum information and alternative reference are not both specified, the critical values are created such that both the specified Type I and the specified Type II error probability levels are maintained in the design. Otherwise, the critical values are created such that either the specified Type I error probability or the specified Type II error probability is maintained.
One-Sided Tests
For a K-stage group sequential design with an upper alternative hypothesis 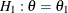 and early stopping to reject or accept the null hypothesis
and early stopping to reject or accept the null hypothesis 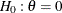 , the boundaries contain the upper
, the boundaries contain the upper  critical values
critical values 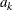 and upper
and upper 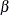 critical values
critical values  ,
,  . At each interim stage,
. At each interim stage,  , the null hypothesis
, the null hypothesis 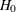 is rejected if the observed statistic
is rejected if the observed statistic 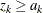 ,
, 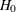 is accepted if
is accepted if  , or the process is continued to the next stage if
, or the process is continued to the next stage if 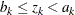 . At the final stage
. At the final stage  , the hypothesis is either rejected or accepted.
, the hypothesis is either rejected or accepted.
The overall Type I error probability  is given by
is given by
![\[ \alpha = \sum _{k=1}^{K} {\alpha _{k}} \]](images/statug_seqdesign0393.png)
where 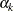 is the
is the  spending at stage k. That is, at stage 1,
spending at stage k. That is, at stage 1,
![\[ {\alpha _{1}} = P_{\theta =0} ( \, z_{1} \geq a_{1} \, ) \]](images/statug_seqdesign0395.png)
At a subsequent stage k,
![\[ {\alpha _{k}} = P_{\theta =0} ( \, b_{j} \leq z_{j} < a_{j}, j=1, 2, \ldots , k-1, \, z_{k} \geq a_{k}) \]](images/statug_seqdesign0396.png)
Similarly, the Type II error probability
![\[ \beta = \sum _{k=1}^{K} {\beta _{k}} \]](images/statug_seqdesign0397.png)
where 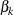 is the
is the 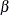 spending at stage k. That is, at stage 1,
spending at stage k. That is, at stage 1,
![\[ {\beta _{1}} = P_{\theta =\theta _1} ( \, z_{1} < b_{1} \, ) \]](images/statug_seqdesign0399.png)
At a subsequent stage k,
![\[ {\beta _{k}} = P_{\theta =\theta _1} ( \, b_{j} \leq z_{j} < a_{j}, j=1, 2, \ldots , k-1, \, z_{k} < b_{k} \, ) \]](images/statug_seqdesign0400.png)
With an upper alternative hypothesis 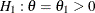 , the power
, the power 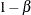 is the probability of rejecting the null hypothesis for the upper alternative.
is the probability of rejecting the null hypothesis for the upper alternative.
![\[ 1-\beta = 1 - \sum _{k=1}^{K} {\beta _{k}} = \sum _{k=1}^{K} P_{\theta =\theta _1} ( \, \, b_{j} \leq z_{j} < a_{j}, j=1, 2, \ldots , k-1, \, z_{k} \geq a_{k}) \]](images/statug_seqdesign0402.png)
For a design with early stopping to reject 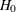 only, the interim upper
only, the interim upper 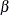 critical values are set to
critical values are set to 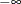 ,
,  , and
, and 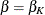 . For a design with early stopping to accept
. For a design with early stopping to accept 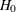 only, the interim upper
only, the interim upper  critical values are set to
critical values are set to 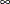 ,
,  , and
, and  .
.
Similarly, the Type I and Type II error probabilities for a K-stage design with a lower alternative hypothesis 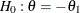 can also be derived.
can also be derived.
Two-Sided Tests
For a K-stage group sequential design with two-sided alternative hypotheses 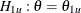 and
and 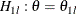 , and early stopping to reject or accept the null hypothesis
, and early stopping to reject or accept the null hypothesis 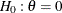 , the boundaries contain the upper
, the boundaries contain the upper  critical values
critical values 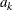 , upper
, upper 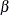 critical values
critical values  , lower
, lower 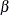 critical values
critical values 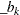 , and lower
, and lower  critical values
critical values 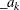 ,
,  . At each interim stage,
. At each interim stage, 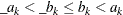 , the null hypothesis
, the null hypothesis 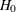 is rejected if the observed statistic
is rejected if the observed statistic 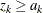 or
or 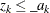 ,
, 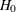 is accepted if
is accepted if 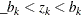 , or the process is continued to the next stage if
, or the process is continued to the next stage if 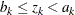 or
or 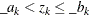 . At the final stage
. At the final stage  and
and  , the hypothesis is either rejected or accepted.
, the hypothesis is either rejected or accepted.
The overall upper Type I error probability 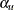 is given by
is given by
![\[ \alpha _{u} = \sum _{k=1}^{K} {\alpha _{uk}} \]](images/statug_seqdesign0419.png)
where 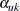 is the
is the  spending at stage k for the upper alternative. That is, at stage 1,
spending at stage k for the upper alternative. That is, at stage 1,
![\[ {\alpha _{u1}} = P_{\theta =0} ( \, z_{1} \geq a_{1} \, ) \]](images/statug_seqdesign0421.png)
At a subsequent stage k,
![\[ {\alpha _{uk}} = P_{\theta =0} ( \, \_ a_{j} < z_{j} \leq \_ b_{j} \, \, \mr{or} \, \, b_{j} \leq z_{j} < a_{j}, j=1, 2, \ldots , k-1, \, z_{k} \geq a_{k} \, ) \]](images/statug_seqdesign0422.png)
Similarly, the overall lower Type I error probability 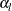 can also be derived, and the overall Type I error probability
can also be derived, and the overall Type I error probability 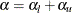 .
.
The overall upper Type II error probability 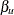 is given by
is given by
![\[ \beta _ u = \sum _{k=1}^{K} {\beta _{uk}} \]](images/statug_seqdesign0424.png)
where  is the upper
is the upper 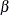 spending at stage k. That is, at stage 1,
spending at stage k. That is, at stage 1,
![\[ {\beta _{u1}} = P_{\theta =\theta _{1u}} ( \, z_{1} < \_ a_{1} \, \, \mr{or} \, \, \_ b_{1} < z_{1} < b_{1} \, ) \]](images/statug_seqdesign0426.png)
At a subsequent stage k,
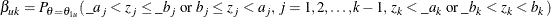
With an upper alternative hypothesis  , the power
, the power 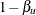 is the probability of rejecting the null hypothesis for the upper alternative:
is the probability of rejecting the null hypothesis for the upper alternative:
![\[ 1-\beta _ u = 1 - \sum _{k=1}^{K} {\beta _{uk}} \]](images/statug_seqdesign0430.png)
which is
![\[ P_{\theta =\theta _{1u}} ( \, \_ a_{j} < z_{j} \leq \_ b_{j} \, \, \mr{or} \, \, b_{j} \leq z_{j} < a_{j}, \, j=1, 2, \ldots , k-1, \, z_{k} \geq a_{k} \, ) \]](images/statug_seqdesign0431.png)
The overall lower Type II error probability 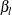 and power
and power 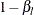 can be similarly derived.
can be similarly derived.
For a design with early stopping only to reject 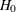 , both the interim lower and upper
, both the interim lower and upper 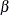 critical values are set to missing,
critical values are set to missing, 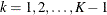 , and
, and 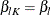 ,
,  . For a design with early stopping only to accept
. For a design with early stopping only to accept 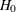 , the interim upper
, the interim upper  critical values are set to
critical values are set to 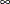 ,
, 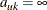 , and the interim lower
, and the interim lower  critical values are set to
critical values are set to 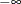 ,
, 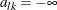 ,
, 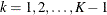 , and
, and 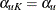 ,
, 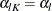 .
.