The SEQDESIGN Procedure

One-Sided Fixed-Sample Tests in Clinical Trials

Subsections:

A one-sided test has either an upper (greater) or a lower (lesser) alternative. This section describes one-sided tests with upper alternatives only. Corresponding results for one-sided tests with lower alternatives can be derived similarly.

For a one-sided test of 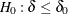$H_{0}: \delta \leq \delta _{0}$ with an upper alternative 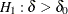$H_{1}: \delta > \delta _{0}$, an equivalent null hypothesis is 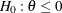$H_{0}: \theta \leq 0$ with an upper alternative 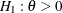$H_{1}: \theta > 0$, where 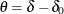$\theta = \delta - \delta _{0}$. A fixed-sample test rejects 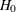$H_{0}$ if the standardized test statistic 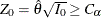$Z_{0} = \hat{\theta } \sqrt {I_{0}} \geq C_{\alpha }$, where 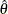$\hat{\theta }$ is the sample estimate of 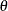$\theta $ and $C_{\alpha } = {\Phi }^{-1}(1-\alpha )$ is the critical value.

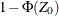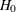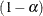The p-value of the test is given by $1-{\Phi }(Z_{0})$, and the hypothesis $H_{0}$ is rejected if the p-value is less than $\alpha $. An upper $(1-\alpha )$ confidence interval has the lower limit

\[ \theta _{l} = \hat{\theta } - \frac{ {\Phi }^{-1}(1-\alpha ) }{ \sqrt {I_{0}} } = \frac{Z_{0} - {\Phi }^{-1}(1-\alpha )}{ \sqrt {I_{0}} } \]

The hypothesis 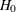$H_{0}$ is rejected if the confidence interval for the parameter 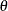$\theta $ does not contain zero—that is, if the lower limit 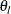$\theta _{l}$ is greater than 0.

With an alternative reference 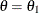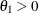$\theta = \theta _1$, $\theta _1 > 0$, a Type II error probability is defined as

\[ \beta = P_{\theta =\theta _1} ( Z_{0} < C_{\alpha } ) \]

which is equivalent to

\[ \beta = P_{\theta =\theta _1} \left( Z_{0} - \theta _1 \sqrt {I_{0}} \, \, < \, \, C_{\alpha } - \theta _1 \sqrt {I_{0}} \right) = {\Phi } \left( C_{\alpha } - \theta _1 \sqrt {I_{0}} \right) \]

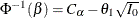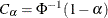Thus, ${\Phi }^{-1}(\beta ) = C_{\alpha } - \theta _1 \sqrt {I_{0}}$. Then, with $C_{\alpha }={\Phi }^{-1}(1-\alpha )$,

\[ \theta _1 \sqrt {I_{0}} = {\Phi }^{-1}(1-\alpha ) + {\Phi }^{-1}(1-\beta ) \]

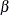The drift parameter $\theta _1 \sqrt {I_{0}}$ can be computed for specified $\alpha $ and $\beta $ and the maximum information is given by

\[ I_{0} = \, \left( \frac{ {\Phi }^{-1}(1-\alpha ) + {\Phi }^{-1}(1-\beta ) }{ \theta _1 } \right)^{2} \]

If the maximum information is available, then the required sample size can be derived. For example, in a one-sample test for the mean with a specific standard deviation $\sigma $, the sample size n required for the test is

\[ n= {\sigma }^{2} \, I_{0} = \, {\sigma }^{2} \left( \frac{ {\Phi }^{-1}(1-\alpha ) + {\Phi }^{-1}(1-\beta ) }{ \theta _1 } \right)^{2} \]

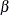On the other hand, if the alternative reference ${\theta }_1$, standard deviation $\sigma $, and sample size n are all specified, then $\alpha $ can be derived for a given $\beta $ and, similarly, 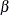$\beta $ can be derived for a given $\alpha $.

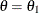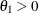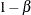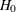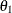With an alternative reference $\theta = \theta _1$, $\theta _1 > 0$, the power $1-\beta $ is the probability of correctly rejecting the null hypothesis $H_0$ at $\theta _1$:

\[ 1 - \beta = 1 - P_{\theta =\theta _1} ( Z_{0} < C_{\alpha } ) = {\Phi } \left( \theta _1 \sqrt {I_{0}} - C_{\alpha } \right) \]

Superiority Trials

A superiority trial that tests the response to a new drug is clinically superior to a comparative placebo control or active control therapy. If a positive value indicates a beneficial effect, a test for superiority has

\[ H_{0}: \theta \leq 0 \, \, \, \, \, \, \, \, H_{1}: \theta > 0 \]

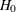where $H_{0}$ is the hypothesis of nonsuperiority and 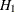$H_{1}$ is the alternative hypothesis of superiority.

The superiority test rejects the hypothesis 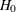$H_{0}$ and declares superiority if the standardized statistic 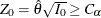$Z_{0}= \hat{\theta } \sqrt {I_{0}} \geq C_{\alpha }$, where the critical value $C_{\alpha } = {\Phi }^{-1}(1-\alpha )$.

For example, if 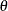$\theta $ is the response difference between the treatment and placebo control groups, then a superiority trial can be

\[ H_{0}: \theta \leq 0 \, \, \, \, \, \, \, \, H_{1}: \theta = 6 \]

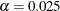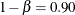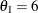with a Type I error probability level $\alpha =0.025$ and a power $1-\beta = 0.90$ at $\theta _1= 6$.

Noninferiority Trials

A noninferiority trial does not compare the response to a new treatment with the response to a placebo. Instead, it demonstrates the effectiveness of a new treatment compared with that of a nonexisting placebo by showing that the response of a new treatment is not clinically inferior to the response of a standard therapy with an established effect. That is, this type of trial attempts to demonstrate that the new treatment effect is not worse than the standard therapy effect by an acceptable margin. These trials are often performed when there is an existing effective therapy for a serious disease, and therefore a placebo control group cannot be ethically included.

It can be difficult to specify an appropriate noninferiority margin. One practice is to choose with reference to the effect of the active control in historical placebo-controlled trials (Snapinn 2000, p. 20). With this practice, there is some basis to imply that the new treatment is better than the placebo for a positive noninferiority trial.

If a positive value indicates a beneficial effect, a test for noninferiority has a null hypothesis 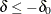$\delta \leq -\delta _{0}$ and an alternative hypothesis $\delta = \delta _{1} > -\delta _{0}$, where $\delta _{0} > 0$ is the specified noninferiority margin.

An equivalent test has

\[ H_{0}: \theta \leq 0 \, \, \, \, \, \, \, \, H_{1}: \theta = \theta _1 > 0 \]

where the parameter 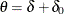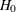$\theta = \delta + \delta _{0}$, $H_{0}$ is the null hypothesis of inferiority, and 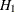$H_{1}$ is the alternative hypothesis of noninferiority,

The noninferiority test rejects the hypothesis 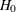$H_{0}$ and declares noninferiority if the standardized statistic $Z_{0}= \hat{\theta } \sqrt {I_{0}} = (\hat{\delta } + \delta _{0}) \sqrt {I_{0}} \geq C_{\alpha }$, where the critical value $C_{\alpha } = {\Phi }^{-1}(1-\alpha )$.

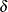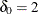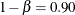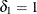For example, if $\delta $ is the response difference between the treatment and active control groups and $\delta _{0}=2$ is the noninferiority margin, then a noninferiority trial with a power $1-\beta = 0.90$ at $\delta _{1}= 1$ might be

\[ H_{0}: \theta \leq 0 \, \, \, \, \, \, \, \, H_{1}: \theta = 3 \]

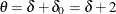where $\theta = \delta + \delta _{0}= \delta + 2$.