The UNIVARIATE Procedure
- Overview
-
Getting Started

-
Syntax

-
Details
 Missing ValuesRoundingDescriptive StatisticsCalculating the ModeCalculating PercentilesTests for LocationConfidence Limits for Parameters of the Normal DistributionRobust EstimatorsCreating Line Printer PlotsCreating High-Resolution GraphicsUsing the CLASS Statement to Create Comparative PlotsPositioning InsetsFormulas for Fitted Continuous DistributionsGoodness-of-Fit TestsKernel Density EstimatesConstruction of Quantile-Quantile and Probability PlotsInterpretation of Quantile-Quantile and Probability PlotsDistributions for Probability and Q-Q PlotsEstimating Shape Parameters Using Q-Q PlotsEstimating Location and Scale Parameters Using Q-Q PlotsEstimating Percentiles Using Q-Q PlotsInput Data SetsOUT= Output Data Set in the OUTPUT StatementOUTHISTOGRAM= Output Data SetOUTKERNEL= Output Data SetOUTTABLE= Output Data SetTables for Summary StatisticsODS Table NamesODS Tables for Fitted DistributionsODS GraphicsComputational Resources
Missing ValuesRoundingDescriptive StatisticsCalculating the ModeCalculating PercentilesTests for LocationConfidence Limits for Parameters of the Normal DistributionRobust EstimatorsCreating Line Printer PlotsCreating High-Resolution GraphicsUsing the CLASS Statement to Create Comparative PlotsPositioning InsetsFormulas for Fitted Continuous DistributionsGoodness-of-Fit TestsKernel Density EstimatesConstruction of Quantile-Quantile and Probability PlotsInterpretation of Quantile-Quantile and Probability PlotsDistributions for Probability and Q-Q PlotsEstimating Shape Parameters Using Q-Q PlotsEstimating Location and Scale Parameters Using Q-Q PlotsEstimating Percentiles Using Q-Q PlotsInput Data SetsOUT= Output Data Set in the OUTPUT StatementOUTHISTOGRAM= Output Data SetOUTKERNEL= Output Data SetOUTTABLE= Output Data SetTables for Summary StatisticsODS Table NamesODS Tables for Fitted DistributionsODS GraphicsComputational Resources -
Examples
 Computing Descriptive Statistics for Multiple VariablesCalculating ModesIdentifying Extreme Observations and Extreme ValuesCreating a Frequency TableCreating Plots for Line Printer OutputAnalyzing a Data Set With a FREQ VariableSaving Summary Statistics in an OUT= Output Data SetSaving Percentiles in an Output Data SetComputing Confidence Limits for the Mean, Standard Deviation, and VarianceComputing Confidence Limits for Quantiles and PercentilesComputing Robust EstimatesTesting for LocationPerforming a Sign Test Using Paired DataCreating a HistogramCreating a One-Way Comparative HistogramCreating a Two-Way Comparative HistogramAdding Insets with Descriptive StatisticsBinning a HistogramAdding a Normal Curve to a HistogramAdding Fitted Normal Curves to a Comparative HistogramFitting a Beta CurveFitting Lognormal, Weibull, and Gamma CurvesComputing Kernel Density EstimatesFitting a Three-Parameter Lognormal CurveAnnotating a Folded Normal CurveCreating Lognormal Probability PlotsCreating a Histogram to Display Lognormal FitCreating a Normal Quantile PlotAdding a Distribution Reference LineInterpreting a Normal Quantile PlotEstimating Three Parameters from Lognormal Quantile PlotsEstimating Percentiles from Lognormal Quantile PlotsEstimating Parameters from Lognormal Quantile PlotsComparing Weibull Quantile PlotsCreating a Cumulative Distribution PlotCreating a P-P Plot
Computing Descriptive Statistics for Multiple VariablesCalculating ModesIdentifying Extreme Observations and Extreme ValuesCreating a Frequency TableCreating Plots for Line Printer OutputAnalyzing a Data Set With a FREQ VariableSaving Summary Statistics in an OUT= Output Data SetSaving Percentiles in an Output Data SetComputing Confidence Limits for the Mean, Standard Deviation, and VarianceComputing Confidence Limits for Quantiles and PercentilesComputing Robust EstimatesTesting for LocationPerforming a Sign Test Using Paired DataCreating a HistogramCreating a One-Way Comparative HistogramCreating a Two-Way Comparative HistogramAdding Insets with Descriptive StatisticsBinning a HistogramAdding a Normal Curve to a HistogramAdding Fitted Normal Curves to a Comparative HistogramFitting a Beta CurveFitting Lognormal, Weibull, and Gamma CurvesComputing Kernel Density EstimatesFitting a Three-Parameter Lognormal CurveAnnotating a Folded Normal CurveCreating Lognormal Probability PlotsCreating a Histogram to Display Lognormal FitCreating a Normal Quantile PlotAdding a Distribution Reference LineInterpreting a Normal Quantile PlotEstimating Three Parameters from Lognormal Quantile PlotsEstimating Percentiles from Lognormal Quantile PlotsEstimating Parameters from Lognormal Quantile PlotsComparing Weibull Quantile PlotsCreating a Cumulative Distribution PlotCreating a P-P Plot - References
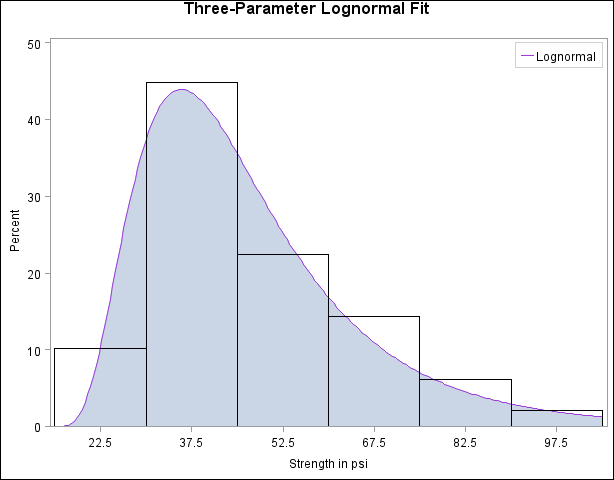
Example 4.24 Fitting a Three-Parameter Lognormal Curve
If you request a lognormal fit with the LOGNORMAL primary option, a two-parameter lognormal distribution is assumed. This
means that the shape parameter ![]() and the scale parameter
and the scale parameter ![]() are unknown (unless specified) and that the threshold
are unknown (unless specified) and that the threshold ![]() is known (it is either specified with the THETA= option or assumed to be zero).
is known (it is either specified with the THETA= option or assumed to be zero).
If it is necessary to estimate ![]() in addition to
in addition to ![]() and
and ![]() , the distribution is referred to as a three-parameter lognormal distribution. This example shows how you can request a three-parameter
lognormal distribution.
, the distribution is referred to as a three-parameter lognormal distribution. This example shows how you can request a three-parameter
lognormal distribution.
A manufacturing process produces a plastic laminate whose strength must exceed a minimum of 25 pounds per square inch (psi).
Samples are tested, and a lognormal distribution is observed for the strengths. It is important to estimate ![]() to determine whether the process meets the strength requirement. The following statements save the strengths for 49 samples
in the data set
to determine whether the process meets the strength requirement. The following statements save the strengths for 49 samples
in the data set Plastic:
data Plastic; label Strength = 'Strength in psi'; input Strength @@; datalines; 30.26 31.23 71.96 47.39 33.93 76.15 42.21 81.37 78.48 72.65 61.63 34.90 24.83 68.93 43.27 41.76 57.24 23.80 34.03 33.38 21.87 31.29 32.48 51.54 44.06 42.66 47.98 33.73 25.80 29.95 60.89 55.33 39.44 34.50 73.51 43.41 54.67 99.43 50.76 48.81 31.86 33.88 35.57 60.41 54.92 35.66 59.30 41.96 45.32 ;
The following statements use the LOGNORMAL primary option in the HISTOGRAM statement to display the fitted three-parameter lognormal curve shown in Output 4.24.1:
title 'Three-Parameter Lognormal Fit'; ods graphics off; proc univariate data=Plastic noprint; histogram Strength / lognormal(fill theta = est noprint); inset lognormal / format=6.2 pos=ne; run;
The NOPRINT option suppresses the tables of statistical output produced by default. Specifying THETA=EST requests a local
maximum likelihood estimate (LMLE) for ![]() , as described by Cohen (1951). This estimate is then used to compute maximum likelihood estimates for
, as described by Cohen (1951). This estimate is then used to compute maximum likelihood estimates for ![]() and
and ![]() .
.
Note: You can also specify THETA=EST with the WEIBULL primary option to fit a three-parameter Weibull distribution.
A sample program for this example, uniex14.sas, is available in the SAS Sample Library for Base SAS software.
Output 4.24.1: Three-Parameter Lognormal Fit