The GLIMMIX Procedure
-
Overview
-
Getting Started
-
Syntax
PROC GLIMMIX Statement BY Statement CLASS Statement CONTRAST Statement COVTEST Statement EFFECT Statement ESTIMATE Statement FREQ Statement ID Statement LSMEANS Statement LSMESTIMATE Statement MODEL Statement NLOPTIONS Statement OUTPUT Statement PARMS Statement RANDOM Statement SLICE Statement STORE Statement WEIGHT Statement Programming Statements User-Defined Link or Variance Function
-
Details
Generalized Linear Models Theory Generalized Linear Mixed Models Theory GLM Mode or GLMM Mode Statistical Inference for Covariance Parameters Satterthwaite Degrees of Freedom Approximation Empirical Covariance (Sandwich) Estimators Exploring and Comparing Covariance Matrices Processing by Subjects Radial Smoothing Based on Mixed Models Odds and Odds Ratio Estimation Parameterization of Generalized Linear Mixed Models Response-Level Ordering and Referencing Comparing the GLIMMIX and MIXED Procedures Singly or Doubly Iterative Fitting Default Estimation Techniques Default Output Notes on Output Statistics ODS Table Names ODS Graphics
-
Examples
Binomial Counts in Randomized Blocks Mating Experiment with Crossed Random Effects Smoothing Disease Rates; Standardized Mortality Ratios Quasi-likelihood Estimation for Proportions with Unknown Distribution Joint Modeling of Binary and Count Data Radial Smoothing of Repeated Measures Data Isotonic Contrasts for Ordered Alternatives Adjusted Covariance Matrices of Fixed Effects Testing Equality of Covariance and Correlation Matrices Multiple Trends Correspond to Multiple Extrema in Profile Likelihoods Maximum Likelihood in Proportional Odds Model with Random Effects Fitting a Marginal (GEE-Type) Model Response Surface Comparisons with Multiplicity Adjustments Generalized Poisson Mixed Model for Overdispersed Count Data Comparing Multiple B-Splines Diallel Experiment with Multimember Random Effects Linear Inference Based on Summary Data
- References
The Likelihood Ratio Test
The likelihood ratio test (LRT) compares the likelihoods of two models where parameter estimates are obtained in two parameter spaces, the space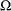 
and the restricted subspace 
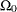. In the GLIMMIX procedure, the full model defines 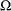and the test-specification in the COVTEST statement determines the null parameter space 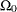. The likelihood ratio procedure consists of the following steps (see, for example, Bickel and Doksum 1977, p. 210):
Find the estimate
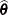of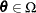 
. Compute the likelihood 
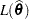. Find the estimate
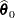of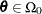 
. Compute the likelihood 
. -
Form the likelihood ratio
Find a function
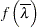that has a known distribution. 
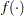serves as the test statistic for the likelihood ratio test.
Please note the following regarding the implementation of these steps in the COVTEST statement of the GLIMMIX procedure.
-
The function
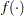in step 4 is always taken to be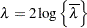 
which is twice the difference between the log likelihoods for the full model and the model under the COVTEST restriction.
For METHOD=RSPL and METHOD=RMPL, the test statistic is based on the restricted likelihood.
For GLMMs involving pseudo-data, the test statistics are based on the pseudo-likelihood or the restricted pseudo-likelihood and are based on the final pseudo-data.
The parameter space
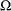for the full model is typically not an unrestricted space. The GLIMMIX procedure imposes boundary constraints for variance components and scale parameters, for example. The specification of the subspace 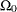must be consistent with these full-model constraints; otherwise the test statistic 
does not have the needed distribution. You can remove the boundary restrictions with the NOBOUND option in the PROC GLIMMIX statement or the NOBOUND option in the PARMS statement.