The SURVEYPHREG Procedure
- Overview
- Getting Started
-
Syntax
PROC SURVEYPHREG Statement BY Statement CLASS Statement CLUSTER Statement DOMAIN Statement ESTIMATE Statement FREQ Statement LSMEANS Statement LSMESTIMATE Statement MODEL Statement NLOPTIONS Statement OUTPUT Statement Programming Statements REPWEIGHTS Statement SLICE Statement STORE Statement STRATA Statement TEST Statement WEIGHT Statement -
Details
Notation and Estimation Failure Time Distribution Time and CLASS Variables Usage Partial Likelihood Function for the Cox Model Specifying the Sample Design Missing Values Variance Estimation Domain Analysis Hypothesis Tests, Confidence Intervals, and Residuals Output Data Sets Displayed Output ODS Table Names ODS Graphics
-
Examples
- References
Contrasts
For a testable hypothesis 
, the Wald F statistic is computed as
 |
where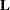 
is a contrast vector or matrix that you specify, 
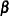is the vector of regression parameters, 
is the estimated regression coefficients,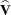 
is the estimated covariance matrix of , rank(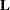) is the rank of 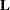, and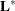 
is a matrix such that
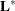- has the same number of columns as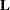
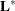- has full row rank
the rank of
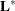equals the rank of the 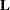matrix all rows of
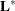are estimable functions the Wald F statistic computed by using the
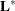matrix is equivalent to the Wald F statistic computed by using the 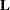matrix with any row deleted that is a linear combination of previous rows
If 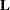is a full-rank matrix and all rows of 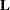are estimable functions, then 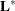is the same as 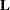. It is possible that 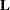matrix cannot be constructed for a given set of linear contrasts, in which case the contrasts are not testable.
If the DF=NONE option in the MODEL statement is specified, then the procedure performs a chi-square significance test.