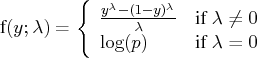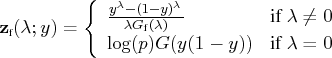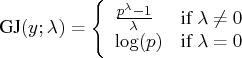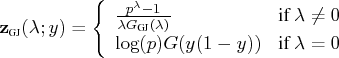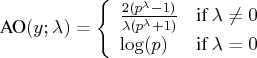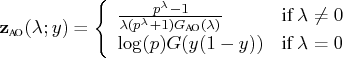Variable Transformations

Transformations for Proportion Variables

Figure 32.14 shows the transformations that are available when you select For proportions from the Family list. These transformations are intended for variables that represent proportions. That is, the variable must take values between 0 and 1. You can also use these transformations for percentages if you first divide the percentages by 100.

Chapter 7 of Atkinson (1985) is devoted to transformations of proportions. Equations for these transformations are given in Table 32.4.

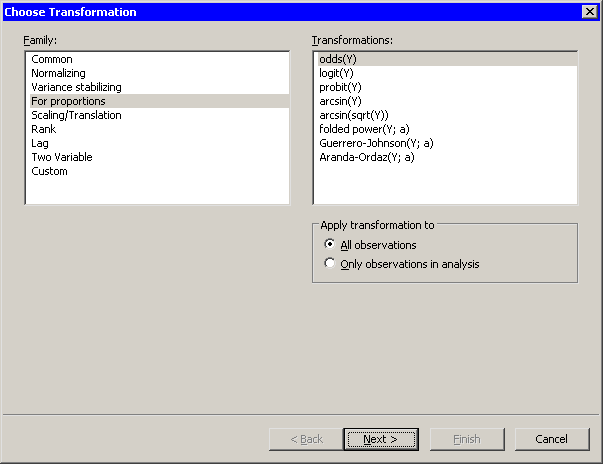Figure 32.14: Transformations for Proportions

Table 32.4: Description of Transformations for Proportions $y\in [0,1)$

	Default	Name of
Transformation	Parameter	New Variable	Equation
odds(Y)		Odds_Y
logit(Y)		Logit_Y	$\log(y/(1-y))$
probit(Y)		Probit_Y
arcsin(Y)		Arcsin_Y	$\arcsin(y)$
arcsin(sqrt(Y))		Angular_Y	$\arcsin(\sqrt{y})$
folded power(Y;a)	MLE	FPow_Y	See text.
Guerrero-Johnson(Y;a)	MLE	GJ_Y	See text.
Aranda-Ordaz(Y;a)	MLE	AO_Y	See text.

The probit function is the quantile function of the standard normal distribution.

The last three transformations in the list are similar to the Box-Cox transformation described in the section "Normalizing Transformations". The parameter for each transformation is in the unit interval: $a\in[0,1]$ . Typically, you choose a parameter that maximizes (or nearly maximizes) a log-likelihood function.

The log-likelihood function is defined as follows. Let be the number of nonmissing values, and let $g(\cdot)$ be the geometric mean function. Each transformation has a corresponding normalized transformation ${z}(\lambda; y)$ , to be defined later. Define

$r(\lambda;{z}) = {z}'{z}- (\sigma z_i )^2 / n$

and define the log-likelihood function as

$l(\lambda;{z}) = -(n/2) \log(r(\lambda;{z})/(n-1))$

The following sections define the normalized transformation for the folded power, Guerrero-Johnson, and Aranda-Ordaz transformations. In each section, .

The Folded Power Transformation

The folded power transformation is defined as

$f(y;\lambda) = \{ \frac{y^\lambda - (1-y)^\lambda}{\lambda} & {if } \lambda \neq 0 \ \log(p) & {if } \lambda = 0 .$

The normalized folded power transformation is defined as (Atkinson 1985, p. 139)

${z}_{{\tiny f}}(\lambda; y) = \{ \frac{y^{\lambda} - (1-y)^{\lambda}}{\lambda g_... ...lambda)} & {if } \lambda \neq 0 \ \log(p) g(y(1-y)) & {if } \lambda = 0 .$

where $g_{{\tiny f}}(\lambda)= g(y^{\lambda-1} +(1-y)^{\lambda-1})$ . When you select the folded power transformation, a plot of $l(\lambda;{z}_{{\tiny f}})$ appears. You should choose a value close to the MLE value.

The Guerrero-Johnson Transformation

The Guerrero-Johnson transformation is defined as

${gj}(y;\lambda) = \{ \frac{p^\lambda - 1}{\lambda} & {if } \lambda \neq 0 \ \log(p) & {if } \lambda = 0 .$

The normalized Guerrero-Johnson transformation is defined as (Atkinson 1985, p. 145)

${z}_{{\tiny gj}}(\lambda; y) = \{ \frac{p^{\lambda} - 1}{\lambda g_{{\tiny gj}}(\lambda)} & {if } \lambda \neq 0 \ \log(p) g(y(1-y)) & {if } \lambda = 0 .$

where $g_{{\tiny gj}}(\lambda)= g(y^{\lambda-1}/(1-y)^{\lambda+1})$ . When you select the Guerrero-Johnson transformation, a plot of $l(\lambda;{z}_{{\tiny gj}})$ appears. You should choose a value close to the MLE value.

The Aranda-Ordaz Transformation

The Aranda-Ordaz transformation is defined as

${ao}(y;\lambda) = \{ \frac{2 (p^\lambda - 1)}{\lambda (p^\lambda+1)} & {if } \lambda \neq 0 \ \log(p) & {if } \lambda = 0 .$

The normalized Aranda-Ordaz transformation is defined as (Atkinson 1985, p. 149)

${z}_{{\tiny ao}}(\lambda; y) = \{ \frac{p^\lambda - 1} {\lambda (p^\lambda+1) ... ...lambda)} & {if } \lambda \neq 0 \ \log(p) g(y(1-y)) & {if } \lambda = 0 .$

where $g_{{\tiny ao}}(\lambda)= g(2p^{\lambda-1}(1+p)^2/(p^\lambda+1)^2)$ . When you select the Aranda-Ordaz transformation, a plot of $l(\lambda;{z}_{{\tiny ao}})$ appears. You should choose a value close to the MLE value.

Top of Page