The VARIOGRAM Procedure
Interpretation
For Moran’s I coefficient, ![$I>\mr{E}[I]$](images/statug_variogram0287.png) indicates positive autocorrelation. Positive autocorrelation suggests that neighboring values
indicates positive autocorrelation. Positive autocorrelation suggests that neighboring values 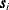 and
and  tend to have similar feature values
tend to have similar feature values  and
and 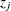 , respectively. When
, respectively. When ![$I<\mr{E}[I]$](images/statug_variogram0288.png) , this is a sign of negative autocorrelation, or dissimilar values at neighboring locations. A measure of strength of the
autocorrelation is the size of the absolute difference
, this is a sign of negative autocorrelation, or dissimilar values at neighboring locations. A measure of strength of the
autocorrelation is the size of the absolute difference ![$\mid I-\mr{E}[I] \mid $](images/statug_variogram0289.png) .
.
Geary’s c coefficient interpretation is analogous to that of Moran’s I. The only difference is that ![$c>\mr{E}[c]$](images/statug_variogram0290.png) indicates negative autocorrelation and dissimilarity, whereas
indicates negative autocorrelation and dissimilarity, whereas ![$c<\mr{E}[c]$](images/statug_variogram0291.png) signifies positive autocorrelation and similarity of values.
signifies positive autocorrelation and similarity of values.
The VARIOGRAM procedure uses the mathematical definitions in the preceding section to provide the observed and expected values, and the standard deviation of the autocorrelation coefficients in the autocorrelation statistics table. The Z scores for each type of statistics are computed as
![\[ Z_ I = \frac{I-\mr{E}[I]}{\sqrt {\mr{Var}[I]}} \]](images/statug_variogram0292.png)
for Moran’s I coefficient, and
![\[ Z_ c = \frac{c-\mr{E}[c]}{\sqrt {\mr{Var}[c]}} \]](images/statug_variogram0293.png)
for Geary’s c coefficient. PROC VARIOGRAM also reports the two-sided p-value for each coefficient under the null hypothesis that the sample values are not autocorrelated. Smaller p-values correspond to stronger autocorrelation for both the I and c statistics. However, the p-value does not tell you whether the autocorrelation is positive or negative. Based on the preceding remarks, you have positive
autocorrelation when  or
or 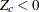 , and you have negative autocorrelation when
, and you have negative autocorrelation when  or
or  .
.
