About the Control Charts Task

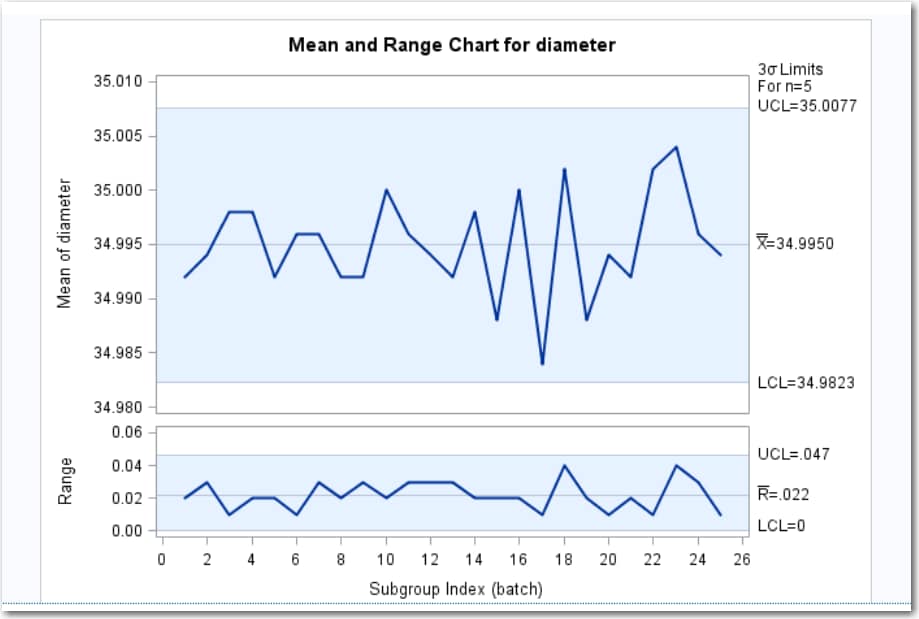
The Mean and Range chart displays the subgroup means and the subgroup ranges. These charts are useful for analyzing the central tendency and the variability of a process.

Suppose that in the manufacture of silicon wafers, batches of five wafers are sampled, and their diameters are measured in millimeters. The measurements for 25 batches are stored in a SAS data set, which is used to create the mean and range charts. Each point on the mean chart represents the average (mean) of the measurements for a particular batch. Each point on the range chart represents the range of the measurements for a particular batch. If all the points fall within the control limits, you can conclude that the process is in statistical control.

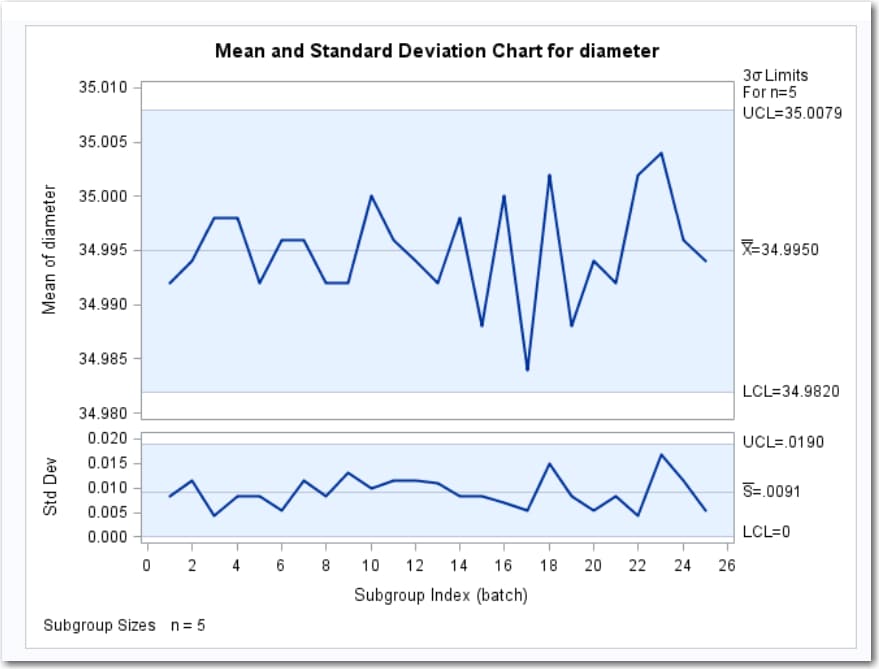
The Mean and Standard Deviation chart displays the subgroup means and the subgroup standard deviations. These charts are useful for analyzing the central tendency and the variability of a process.

You might want to use this chart to find the distribution of the output and to determine whether a process is in statistical control. For example, suppose a petroleum company uses a turbine to heat water into steam that is pumped into the ground to make oil less viscous and easier to extract. This process occurs 20 times daily, and the amount of power (in kilowatts) that is used to heat the water to the desired temperature is recorded. Each point on the mean chart represents the mean of the measurements for a particular day. Each point on the standard deviation chart represents the standard deviation of the measurements for a particular day. If all the points lie within the control limits, it can be concluded that the process is in statistical control.

The Mean with Box-and-Whisker plot is a chart of the subgroup means superimposed with box-and-whisker plots for the measurements in each subgroup.

The Individual Measurements chart displays the individual measurements and the moving ranges. These charts are appropriate when only one measurement is available for each subgroup sample and when the measurements are independently and normally distributed. You might want to use this task to analyze a manufacturing process.

Suppose that an aeronautics company that manufactures jet engines measures the inner diameter of the forward face of each engine (in centimeters). The diameter measurements of 20 engines are stored in a SAS data set. Each point on the individual measurements chart indicates the inner diameter of a particular engine. Each point on the moving range chart indicates the range of the two most recent measurements. If all the individual measurements and moving ranges fall within the control limits, you can conclude that the process is in statistical control.

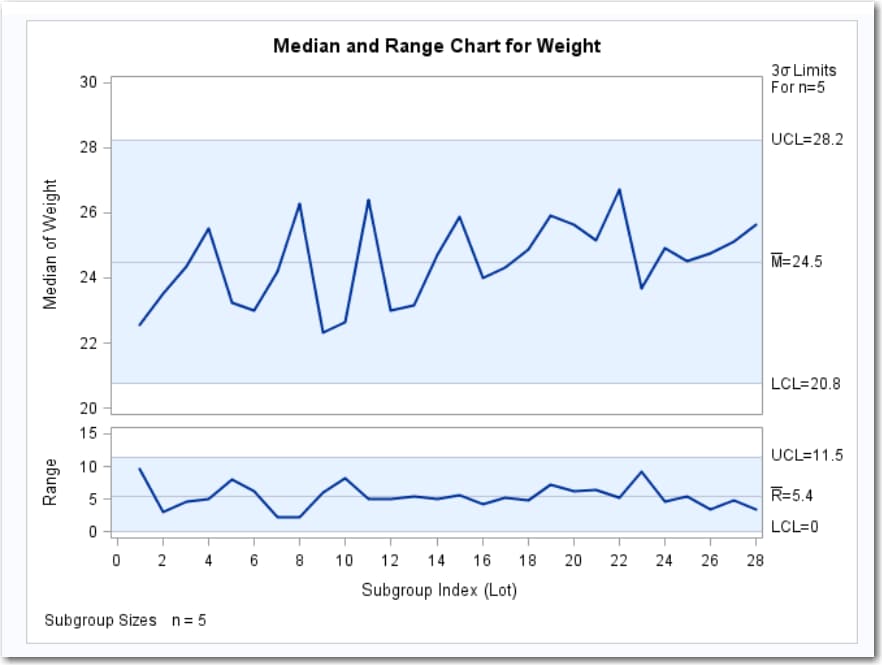
The Median and Range chart displays the subgroup medians and ranges, which are used to analyze the central tendency and variability of a process.

A consumer products company weighs detergent boxes (in pounds) to determine whether the fill process is in control. The Detergent data set contains the weights for five boxes in each of 28 lots. A lot is considered a rational subgroup.

Each point on the median chart represents the median of the measurements for a particular lot. For example, the weights for the first lot are 17.39, 19.34, 22.56, 24.49, and 26.93, and consequently, the median plotted for this lot is 22.56. Each point on the range chart represents the range of the measurements for a particular batch. For example, the range plotted for the first lot is 26.93–17.39=9.54. Because all of the points lie within the control limits, you can conclude that the process is in statistical control.

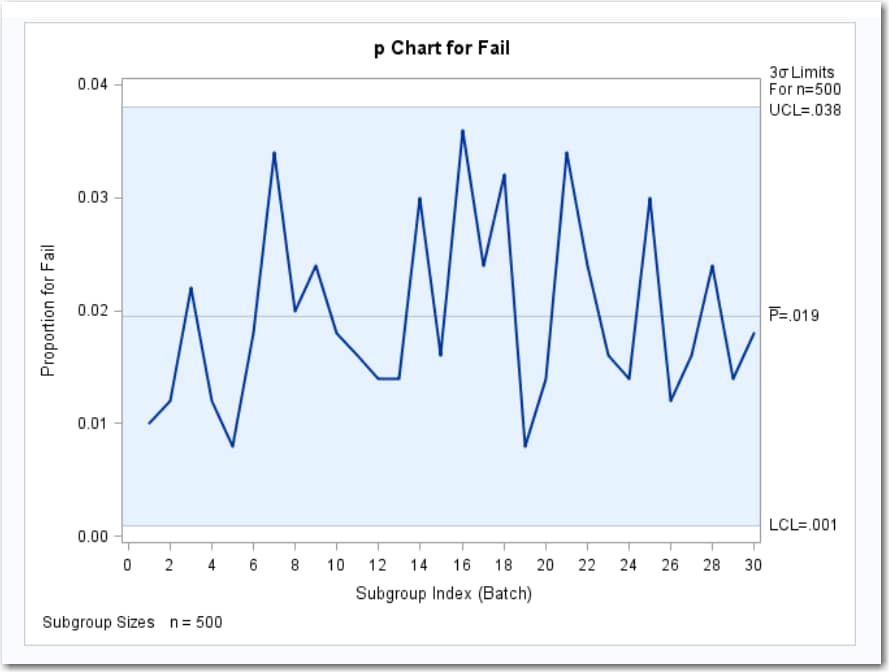
p charts display proportions of nonconforming (defective) items in the subgroup samples. You might want to use this task to monitor the proportion of defects in a manufacturing process.

Suppose that an electronics company manufactures circuits in batches of 500 and uses a p chart to monitor the proportion of failing circuits. Thirty batches are examined, and the failures in each batch are counted. The failure counts are stored in a SAS data set, which is used to create the p chart. Each point on the p chart represents the proportion of nonconforming items in a particular subgroup. For example, if the number of failures in the first circuit is 5, then the value that is plotted for the first batch is 5/500=0.01. If all the points fall within the control limits, it can be concluded that the process is in statistical control.

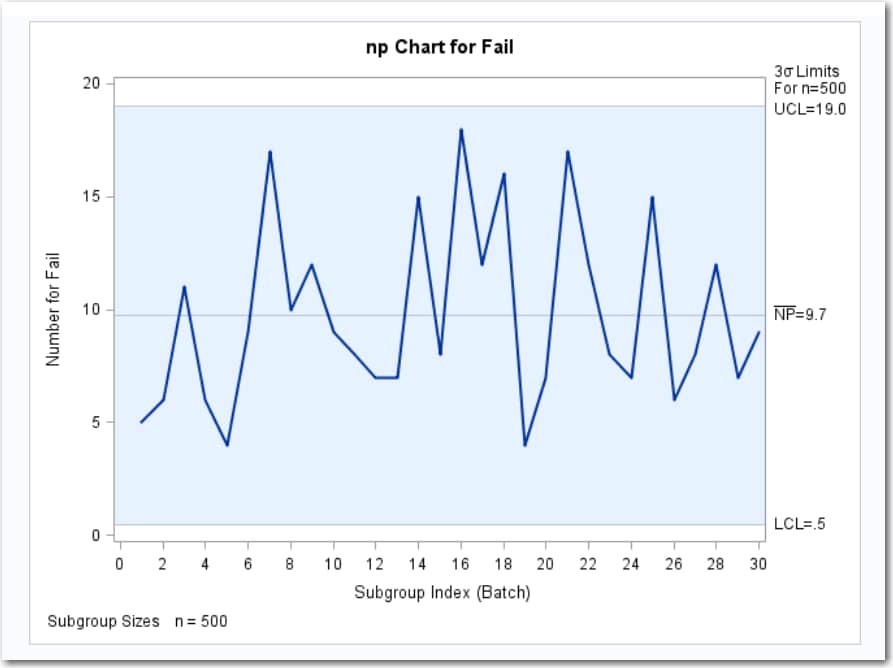
np charts display the numbers of nonconformities (defects) in the subgroup samples. You might want to use this task to monitor the number of defects in a manufacturing process.

Suppose that an electronics company manufactures circuits in batches of 500 and uses an np chart to monitor the number of failing circuits. Thirty batches are examined, and the failures in each batch are counted. The failure counts are stored in a SAS data set, which is used to create the np chart. Each point on the np chart represents the number of nonconforming items in a particular subgroup. For example, the value that is plotted for the first batch is 5. If all the points fall within the control limits, it can be concluded that the process is in statistical control.

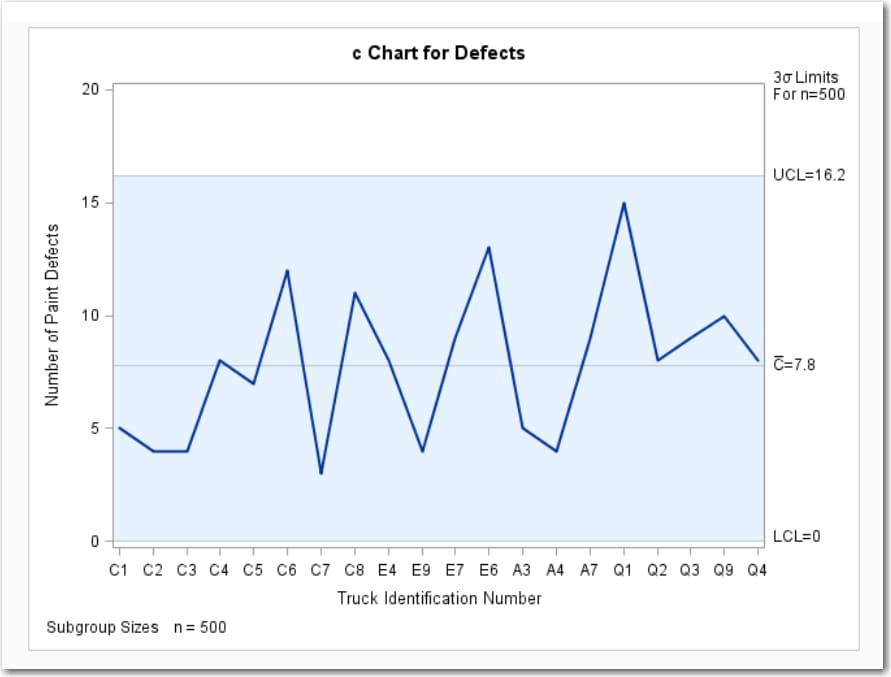
c charts display the numbers of nonconformities (defects) in the subgroup samples. You might want to use a c chart to monitor the number of defects that are found in a new product.

Suppose that an automobile company wants to monitor the number of paint defects on its new trucks. Twenty trucks of the same model are inspected, and the number of paint defects per truck is recorded. Each point on the c chart represents the number of defects for a given truck. If all the points fall within the control limits, it can be concluded that the process is in statistical control.

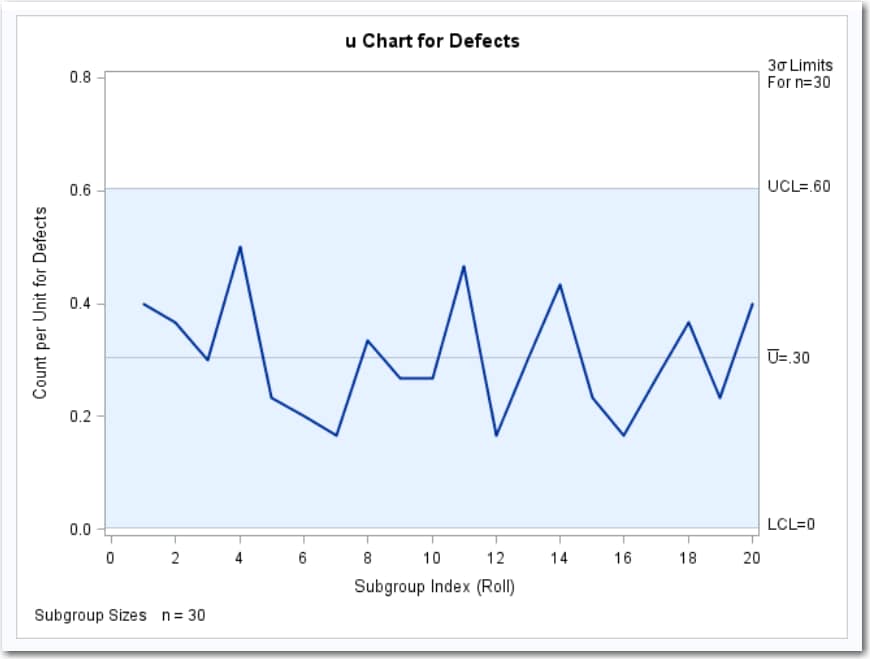
u charts display the numbers of nonconformities (defects) per inspection unit in the subgroup samples that contain arbitrary numbers of units. You might want to use this task to determine the number of defects per inspection unit that resulted from a manufacturing process.

Suppose that a textile company uses a u chart to monitor the number of defects per square meter of fabric. The fabric is spooled onto rolls as it is inspected for defects. Each piece of fabric is one meter wide and 30 meters long. The defect counts for 20 rolls are saved in a SAS data set, which is used to create the u chart. Each point on the u chart represents the number of nonconformities per inspection unit for a particular subgroup. For example, the value that is plotted for the first subgroup is 12/30=0.4 (because there are 12 defects on the first roll and the roll contains 30 square meters of fabric). If none of the points exceed the control limit (which is 3 sigma by default), the u chart indicates that the fabric manufacturing process is in statistical control.