The VARIOGRAM Procedure

Stationarity

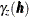In the combined presence of the previous two assumptions—that is, when $\mr{E}[Z(\bm {s})]$ is constant and spatial increments define $\gamma _ z(\bm {h})$—the SRF $Z(\bm {s})$ is characterized as intrinsically stationary (Cressie 1993, p. 40).

The expected value $\mr{E}[Z(\bm {s})]$ is the first statistical moment of the SRF $Z(\bm {s})$. The second statistical moment of the SRF $Z(\bm {s})$ is the covariance function between two points 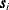$\bm {s}_ i$ and $\bm {s}_ j$ in $Z(\bm {s})$, and it is defined as

\[ C_ z(\bm {s}_ i,\bm {s}_ j) = \mr{E} \left( \left[ Z(\bm {s}_ i)-\mr{E}[Z(\bm {s}_ i)] \right] \left[ Z(\bm {s}_ j)-\mr{E}[Z(\bm {s}_ j)] \right] \right) \]

When $\bm {s}_ i = \bm {s}_ j = \bm {s}$, the covariance expression provides the variance at $\bm {s}$.

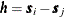The assumption of a constant $\mr{E}[Z(\bm {s})]=m$ means that the expected value is invariant with respect to translations of the spatial location $\bm {s}$. The covariance is considered invariant to such translations when it depends only on the distance $\bm {h}=\bm {s}_ i-\bm {s}_ j$ between any two points 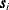$\bm {s}_ i$ and $\bm {s}_ j$. If both of these conditions are true, then the preceding expression becomes

\[ C_ z(\bm {s}_ i,\bm {s}_ j) = C_ z(\bm {s}_ i-\bm {s}_ j) = C_ z(\bm {h}) = \mr{E} \left( [Z(\bm {s})-m][Z(\bm {s}+\bm {h})-m] \right) \]

When both $\mr{E}[Z(\bm {s})]$ and $C(\bm {s}_ i,\bm {s}_ j)$ are invariant to spatial translations, the SRF $Z(\bm {s})$ is characterized as second-order stationary (Cressie 1993, p. 53).

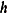In a second-order stationary SRF the quantity $C(\bm {h})$ is the same for any two points that are separated by distance $\bm {h}$. Based on the preceding formula, for 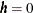$\bm {h}=0$ you can see that the variance is constant throughout a second-order stationary SRF. Hence, second-order stationarity is a stricter condition than intrinsic stationarity.

Under the assumption of second-order stationarity, the semivariance definition at the beginning of this section leads to the conclusion that

\[ \gamma _ z(\bm {h}) = C(\bm {0}) - C(\bm {h}) \]

which relates the theoretical semivariance and covariance. Keep in mind that the empirical estimates of these quantities are not related in exactly the same way, as indicated in Schabenberger and Gotway (2005, section 4.2.1).