The NESTED Procedure

Overview: NESTED Procedure

Subsections:

The NESTED procedure performs random-effects analysis of variance for data from an experiment with a nested (hierarchical) structure.[37] A random-effects model for data from a completely nested design with two factors has the form

\[ y_{ijr} = \mu + \alpha _ i + \beta _{ij} + \epsilon _{ijr} \]

where

$y_{ijr}$

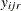
is the value of the dependent variable observed at the rth replication with the first factor at its ith level and the second factor at its jth level.

$\mu $

is the overall (fixed) mean of the sampling population.

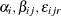
$\alpha _ i,\beta _{ij},\epsilon _{ijr}$

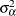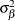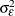are mutually uncorrelated random effects with zero means and respective variances $\sigma _{\alpha }^2$, $\sigma _{\beta }^2$, and $\sigma _{\epsilon }^2$ (the variance components).

This model is appropriate for an experiment with a multistage nested sampling design. An example of this is given in Example 68.1, where four turnip plants are randomly chosen (the first factor), then three leaves are randomly chosen from each plant (the second factor nested within the first), and then two samples are taken from each leaf (the different replications at fixed levels of the two factors).

Note that PROC NESTED is appropriate for models with only classification effects; it does not handle models that contain continuous covariates. For random effects models with covariates, use either the GLM or MIXED procedure.


[37] PROC NESTED is modeled after the General Purpose Nested Analysis of Variance program of the Dairy Cattle Research Branch of the United States Department of Agriculture. That program was originally written by M. R. Swanson, Statistical Reporting Service, United States Department of Agriculture.