The SURVEYREG Procedure
- Overview
-
Getting Started

-
Syntax
 PROC SURVEYREG Statement BY Statement CLASS Statement CLUSTER Statement CONTRAST Statement DOMAIN Statement EFFECT Statement ESTIMATE Statement LSMEANS Statement LSMESTIMATE Statement MODEL Statement OUTPUT Statement REPWEIGHTS Statement SLICE Statement STORE Statement STRATA Statement TEST Statement WEIGHT Statement
PROC SURVEYREG Statement BY Statement CLASS Statement CLUSTER Statement CONTRAST Statement DOMAIN Statement EFFECT Statement ESTIMATE Statement LSMEANS Statement LSMESTIMATE Statement MODEL Statement OUTPUT Statement REPWEIGHTS Statement SLICE Statement STORE Statement STRATA Statement TEST Statement WEIGHT Statement -
Details

-
Examples

- References
Example 90.8 Compare Domain Statistics
This example is a continuation of Example 90.7 in which domain analyses for male and female students were performed. Suppose that you are now interested in estimating the gender domain means of weekly ice cream spending (that is, the average spending for males and females, respectively). You can use the SURVEYMEANS procedure to produce these domain statistics by using the following statements:
proc surveymeans data=IceCreamDataDomain total=StudentTotals; strata Grade; var spending; domain Gender; weight Weight; run;
Output 90.8.1 shows the estimated spending among male and female students.
| Domain Analysis: Gender | ||||||
|---|---|---|---|---|---|---|
| Gender | Variable | N | Mean | Std Error of Mean | 95% CL for Mean | |
| F | Spending | 19 | 9.376111 | 1.077927 | 7.19202418 | 11.5601988 |
| M | Spending | 21 | 8.923052 | 1.003423 | 6.88992385 | 10.9561807 |
You can also use PROC SURVEYREG to estimate these domain means. The benefit of this alternative approach is that PROC SURVEYREG provides more tools for additional analysis, such as domain means comparisons in a LSMEANS statement.
Suppose that you want to test whether there is a significant difference for the ice cream spending between male and female students. You can use the following statements to perform the test:
title1 'Ice Cream Spending Analysis'; title2 'Compare Domain Statistics'; proc surveyreg data=IceCreamDataDomain total=StudentTotals; strata Grade; class Gender; model Spending = Gender / vadjust=none; lsmeans Gender / diff; weight Weight; run;
The variable Gender is used as a model effect. The VADJUST=NONE option is used to produce variance estimates for domain means that are identical to those produced by PROC SURVEYMEANS. The LSMEANS statement requests that PROC SURVEYREG estimate the average spending in each gender group. The DIFF option requests that the procedure compute the difference among domain means.
Output 90.8.2 displays the estimated weekly spending on ice cream among male and female students, respectively, and their standard errors. Female students spend $9.38 per week on average, and male students spend $8.92 per week on average. These domain means, including their standard errors, are identical to those in Output 90.8.1 which are produced by PROC SURVEYMEANS.
| Ice Cream Spending Analysis |
| Compare Domain Statistics |
| Gender Least Squares Means | |||||
|---|---|---|---|---|---|
| Gender | Estimate | Standard Error | DF | t Value | Pr > |t| |
| F | 9.3761 | 1.0779 | 37 | 8.70 | <.0001 |
| M | 8.9231 | 1.0034 | 37 | 8.89 | <.0001 |
Output 90.8.3 shows the estimated difference for weekly ice scream spending between the two gender groups. The female students spend $0.45 more than male students on average, and the difference is not statistically significant based on the t test.
| Differences of Gender Least Squares Means | ||||||
|---|---|---|---|---|---|---|
| Gender | _Gender | Estimate | Standard Error | DF | t Value | Pr > |t| |
| F | M | 0.4531 | 1.7828 | 37 | 0.25 | 0.8008 |
If you want to investigate whether there is any significant difference in ice cream spending among grades, you can use the following similar statements to compare:
title1 'Ice Cream Spending Analysis'; title2 'Compare Domain Statistics'; ods graphics on; proc surveyreg data=IceCreamDataDomain total=StudentTotals; strata Grade; class Grade; model Spending = Grade / vadjust=none; lsmeans Grade / diff plots=(diff meanplot(cl)); weight Weight; run; ods graphics off;
The Grade is specified in the CLASS statement to be used as an effect in the MODEL statement. The DIFF option in the LSMEANS statement requests that the procedure compute the difference among the domain means for the effect Grade. The ODS GRAPHICS statement enables ODS to create graphics. The PLOTS=(DIFF MEANPLOT(CL)) option requests two graphics: the domain means plot "MeanPlot" and their pairwise difference plot "DiffPlot". The CL suboption requests the "MeanPlot" to display confidence. For information about ODS Graphics, see Chapter 21, Statistical Graphics Using ODS.
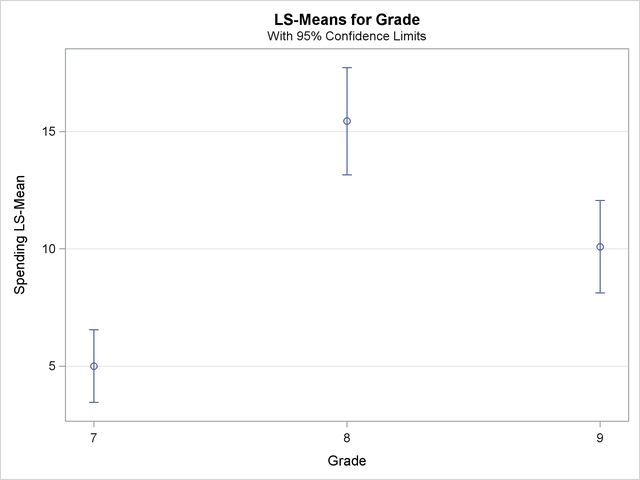
Output 90.8.4 shows the estimated weekly spending on ice cream for students within each grade. Students in Grade 7 spend the least, only $5.00 per week. Students in Grade 8 spend the most, $15.44 per week. Students in Grade 9 spend a little less at $10.09 per week.
| Ice Cream Spending Analysis |
| Compare Domain Statistics |
| Grade Least Squares Means | |||||
|---|---|---|---|---|---|
| Grade | Estimate | Standard Error | DF | t Value | Pr > |t| |
| 7 | 5.0000 | 0.7636 | 37 | 6.55 | <.0001 |
| 8 | 15.4444 | 1.1268 | 37 | 13.71 | <.0001 |
| 9 | 10.0909 | 0.9719 | 37 | 10.38 | <.0001 |
Output 90.8.5 plots the weekly spending results that are shown in Output 90.8.4.
Output 90.8.6 displays pairwise comparisons for weekly ice scream spending among grades. All the differences are significant based on t tests.
| Differences of Grade Least Squares Means | ||||||
|---|---|---|---|---|---|---|
| Grade | _Grade | Estimate | Standard Error | DF | t Value | Pr > |t| |
| 7 | 8 | -10.4444 | 1.3611 | 37 | -7.67 | <.0001 |
| 7 | 9 | -5.0909 | 1.2360 | 37 | -4.12 | 0.0002 |
| 8 | 9 | 5.3535 | 1.4880 | 37 | 3.60 | 0.0009 |
Output 90.8.7 plots the comparisons that are shown in Output 90.8.6.
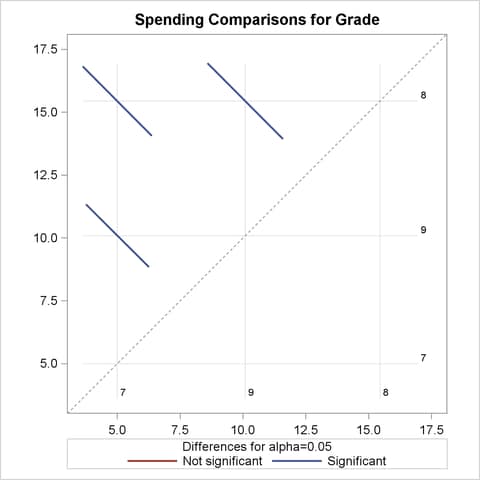
In Output 90.8.7, the spending for each grade is shown in the background grid on both axes. Comparisons for each pair of domain means are shown by colored bars at intersections of these grids. The length of each bar represents the width of the confidence intervals for the corresponding difference between domain means. The significance of these pairwise comparisons are indicated in the plot by whether these bars cross the 45-degree background dash-line across the plot. Since none of the three bars cross the dash-line, all pairwise comparisons are significant, as shown in Output 90.8.6.