PROC MODEL: Testing for Autocorrelation :: SAS/ETS(R) 9.2 User's Guide

The MODEL Procedure

The GODFREY= option in the FIT statement produces the Godfrey Lagrange multiplier test for serially correlated residuals for each equation (Godfrey 1978a and 1978b). $\text{[math]}$ is the maximum autoregressive order, and specifies that Godfrey’s tests be computed for lags 1 through $\text{[math]}$ . The default number of lags is four.

The tests are performed separately for each equation estimated by the FIT statement. When a nonlinear model is estimated, the test is computed by using a linearized model.

The following is an example of the output produced by the GODFREY=3 option:

Figure 18.43 Autocorrelation Test Output

Godfrey Test Output

The MODEL Procedure

Godfrey's Serial Correlation Test
Equation	Alternative	LM	Pr > LM
y	1	6.63	0.0100
	2	6.89	0.0319
	3	6.96	0.0732

The three variations of the test reported by the GODFREY=3 option are designed to have power against different alternative hypothesis. Thus, if the residuals in fact have only first-order autocorrelation, the lag 1 test has the most power for rejecting the null hypothesis of uncorrelated residuals. If the residuals have second- but not higher-order autocorrelation, the lag 2 test might be more likely to reject; the same is true for third-order autocorrelation and the lag 3 test.

The null hypothesis of Godfrey’s tests is that the equation residuals are white noise. 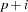However, if the equation includes autoregressive error model of order $\text{[math]}$ (AR( $\text{[math]}$ ),) then the lag $\text{[math]}$ test, when considered in terms of the structural error, is for the null hypothesis that the structural errors are from an AR( $\text{[math]}$ ) process versus the alternative hypothesis that the errors are from an AR( $\text{[math]}$ ) process.

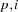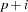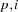The alternative ARMA( $\text{[math]}$ ) process is locally equivalent to the alternative AR( $\text{[math]}$ ) process with respect to the null model AR( $\text{[math]}$ ). Thus, the GODFREY= option results are also a test of AR( $\text{[math]}$ ) errors against the alternative hypothesis of ARMA( $\text{[math]}$ ) errors. See Godfrey (1978a and 1978b) for more detailed information.

Top of Page