Confidence Intervals

Sample Size and Power Calculations

Confidence Intervals

Power calculations are available when the proposed analysis is construction of confidence intervals of a mean (one-sample) or difference of two means (two-samples or paired-samples). To understand the power of a confidence interval, first define the precision to be half the length of a two-sided confidence interval (or the distance between the endpoint and the parameter estimate in a one-sided interval). The power can then be considered to be the probability that the desired precision is achieved, that is, the probability that the length of the two-sided interval is no more than twice the desired precision. Here, a slight modification of this concept is used. The power is considered to be the conditional probability that the desired precision is achieved, given that the interval includes the true value of the parameter of interest. The reason for the modification is that there is no reason to want the interval to be particularly small if it does not contain the true value of the parameter.

To compute the power of a confidence interval or an equivalence test, you make use of Owen's Q formula (Owen 1965). The formula is given by

$Q_{\nu}(t,\delta; a, b)= \frac{\sqrt{2\pi}}{\Gamma(\frac{\nu}2)2^{(\nu-2)/2}} \int_{a}^b\Phi(\frac{tx}{\sqrt{\nu}} - \delta)x^{\nu-1}\phi(x)dx$

where

$\Phi = \int_{-\infty}^x\phi(t)dt$

and

$\phi(x) = \frac{1}{\sqrt{2\pi}}e^{(-x^2/2)}$

The power of a confidence interval (Beal 1989) is given by

${\rm Power} = \frac{2[Q_{\nu}(t_{c}, 0; 0, B) - Q_{\nu}(0, 0; 0, B)]}{1-\alpha_{s}}$

where

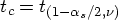
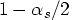$t_c = t_{(1-\alpha_{s}/2,\nu)}$: is the ( $1-\alpha_{s}/2$ ) quantile of a t distribution with 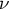$\nu$ df
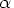
$\alpha$: is the confidence level

$\alpha_s = \{ \alpha & {for a two-sided confidence interval} \ 2\alpha & {for a one-sided confidence interval} .$

$B = \frac{\delta\sqrt{\nu}}{t_c\kappa}$

$. \nu = n-1 \ \kappa = \sqrt{1/n} \} {for the one-sample and paired confidence intervals}$

$. \nu =2(n-1) \ \kappa = \sqrt{2/n} \} {for the two-sample confidence interval}$

$\delta=\frac{\rm desiredprecision}{\rm standarddeviation} { is the upper bound of the interval half-length}$

Top of Page