The VTSROOT subroutine computes the characteristic roots of the model from AR and MA characteristic functions.
The input arguments to the VTSROOT subroutine are as follows:
- phi
-
specifies a
 matrix that contains the autoregressive coefficient matrices, where
matrix that contains the autoregressive coefficient matrices, where 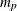 is the number of the elements in the subset of the AR order and
is the number of the elements in the subset of the AR order and 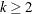 is the number of variables. You must specify either phi or theta.
is the number of variables. You must specify either phi or theta.
- theta
-
specifies a
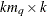 matrix that contains the moving average coefficient matrices, where
matrix that contains the moving average coefficient matrices, where 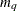 is the number of the elements in the subset of the MA order. You must specify either phi or theta.
is the number of the elements in the subset of the MA order. You must specify either phi or theta.
- p
-
specifies the subset of the AR order. See the VARMACOV subroutine.
- q
-
specifies the subset of the MA order. See the VARMACOV subroutine.
The VTSROOT subroutine returns the following value:
- root
-
is a
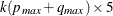 matrix, where
matrix, where 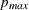 is the maximum order of the AR characteristic function and
is the maximum order of the AR characteristic function and 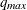 is the maximum order of the MA characteristic function. The first
is the maximum order of the MA characteristic function. The first 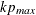 rows refer to the results of the AR characteristic function; the last
rows refer to the results of the AR characteristic function; the last 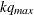 rows refer to the results of the MA characteristic function.
rows refer to the results of the MA characteristic function.
The first column contains the real parts,
 , of eigenvalues of companion matrix associated with the AR(
, of eigenvalues of companion matrix associated with the AR(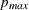 ) or MA(
) or MA(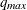 ) characteristic function; the second column contains the imaginary parts,
) characteristic function; the second column contains the imaginary parts,  , of the eigenvalues; the third column contains the moduli of the eigenvalues,
, of the eigenvalues; the third column contains the moduli of the eigenvalues,  ; the fourth column contains the arguments (
; the fourth column contains the arguments (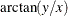 ) of the eigenvalues, measured in radians from the positive real axis. The fifth column contains the arguments expressed in
degrees rather than radians.
) of the eigenvalues, measured in radians from the positive real axis. The fifth column contains the arguments expressed in
degrees rather than radians.
Consider the roots of the characteristic functions, ![]() and
and ![]() , where
, where ![]() is an identity matrix with dimension 2 and
is an identity matrix with dimension 2 and
To compute these roots, you can use the following statements:
phi = { 1.2 -0.5, 0.6 0.3 };
theta= {-0.6 0.3, 0.3 0.6 };
call vtsroot(root, phi, theta);
cols = {"Real" "Imag" "Modulus" "Radians" "Degrees"};
print root[colname=cols];
Figure 24.429: Characteristic Roots
| root | ||||
|---|---|---|---|---|
| Real | Imag | Modulus | Radians | Degrees |
| 0.75 | 0.3122499 | 0.8124038 | 0.3945069 | 22.603583 |
| 0.75 | -0.31225 | 0.8124038 | -0.394507 | -22.60358 |
| 0.6708204 | 0 | 0.6708204 | 0 | 0 |
| -0.67082 | 0 | 0.6708204 | 3.1415927 | 180 |