One-Way ANOVA

Sample Size and Power Calculations

One-Way ANOVA

The power for the one-way ANOVA is computed in a similar manner as for the hypothesis tests. That is, power is the probability of correctly rejecting the null (all group means are equal) in favor of the alternative hypothesis (at least one group mean is not equal), when the alternative is true. The sample size is the number per group; these calculations assume equally sized groups. To compute the power, you make use of the noncentral F distribution. The formula (O'Brien and Lohr 1984) is given by

${Power} = {Prob}(F \gt F_{crit}, \nu_1, \nu_2, NC)$

where F is distributed as the noncentral $F(NC,\nu_1,\nu_2)$ and $F_{crit} = F_{(1-\alpha,\nu_1,\nu_2)}$ is the ( $1-\alpha$ ) quantile of the F distribution with ${\nu_1}$ and ${\nu_2}$ degrees of freedom.

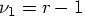
$\nu_1 = r - 1$: is the numerator df
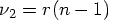
$\nu_2 = r(n-1)$: is the denominator df
n: is the number per group
r: is the number of groups
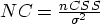
$NC = \frac{n CSS}{\sigma^2}$: is the noncentrality parameter
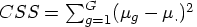
$CSS = \sum_{g=1}^G (\mu_g - \mu_.)^2$: is the corrected sum of squares
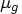
$\mu_g$: is the mean of the gth group
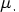
$\mu_.$: is the overall mean
$\sigma^2$: is estimated by the mean squared error (MSE)

Top of Page