Sample Size and Power Calculations

Confidence Intervals

Sample size and power calculations are available for one-sample and two-sample paired and independent designs, when the proposed analysis is construction of confidence intervals of a mean (one-sample) or difference of two means (two-sample), via the t-test.

To understand the power of a confidence interval, first define the precision to be half the length of a two-sided confidence interval (or the distance between the endpoint and the parameter estimate in a one-sided interval). The power can then be considered to be the probability that the desired precision is achieved, that is, the probability that the length of the two-sided interval is no more than twice the desired precision. Here, a slight modification of this concept is used. The power is considered to be the conditional probability that the desired precision is achieved, given that the interval includes the true value of the parameter of interest. The reason for the modification is that there is no reason for the interval to be particularly small if it does not contain the true value of the parameter.

These computations assume equally sized groups.

Requesting Power Computations for a Confidence Interval in a Paired t-test

To perform this task, select

Statistics

Sample Size

Paired Confidence Interval ...

Figure 12.6 displays the resulting dialog.

Figure 12.6: Sample Size Dialog for Paired Confidence Interval

You specify whether you want to compute sample sizes or power, enter values for desired precision and standard deviation, enter the alpha levels, enter the sample sizes or power, and select if you want a power curve.

To request power for a paired confidence interval where the desired precision is 0.5 and the standard deviation is 2.462, follow these steps:

Select Power.
Enter 0.5 as the Desired precision:
Enter 2.462 as the Std dev of diff:
Enter 0.01, 0.025, 0.05, and 0.1 as values in the field for Alpha:
Enter 11 as the value for the From: field in the line for N:
Enter 211 and 5 as the values under To: and By:, respectively, in the line for N:
Select Power vs. N.
Select 2-sided for Interval if it is not already selected.

Note that you can enter multiple values in these fields, for example, for Alpha: and Desired precision:, separated by commas or blanks, and the analysis will be performed for all combinations of the input values. Here, power will be computed for sample sizes ranging from 11 to 211 in multiples of 5.

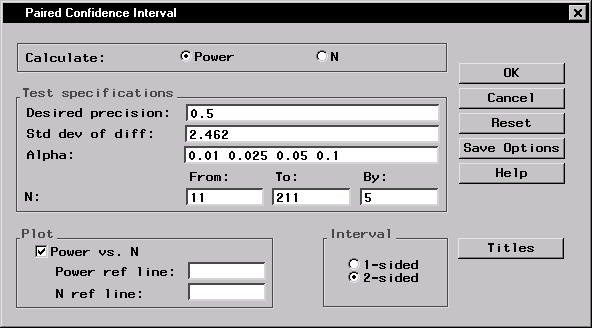Figure 12.7: Completed Sample Size Dialog for Paired Confidence Interval

Figure 12.7 contains the completed dialog. Note that, because multiple alpha values were entered, sets of results will be created for each one.

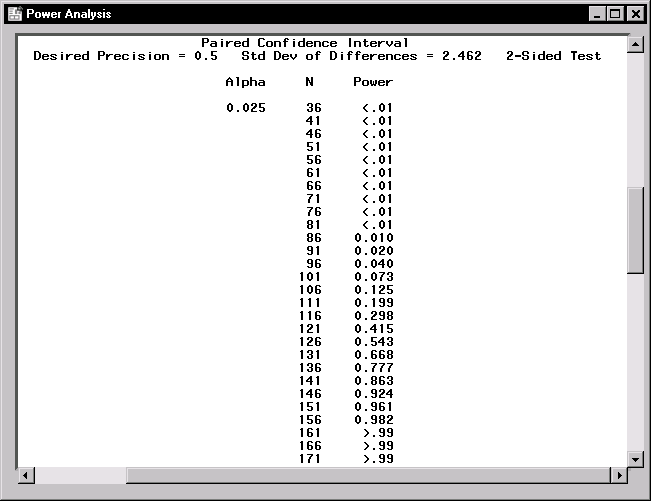Figure 12.8: Sample Size Results for Paired Confidence Interval

Figure 12.8 contains the power computations for the sample sizes ranging from 36 to 171. The power analysis results in Figure 12.8 show that, for a two-sided paired confidence interval where the standard deviation of the differences is 2.462, the significance level is $\alpha$ = 0.025, the sample size is 121, and the power is 0.415. That is, 0.415 represents the probability that a confidence interval containing the true parameter value has a length of no more than twice the desired precision of 0.5.

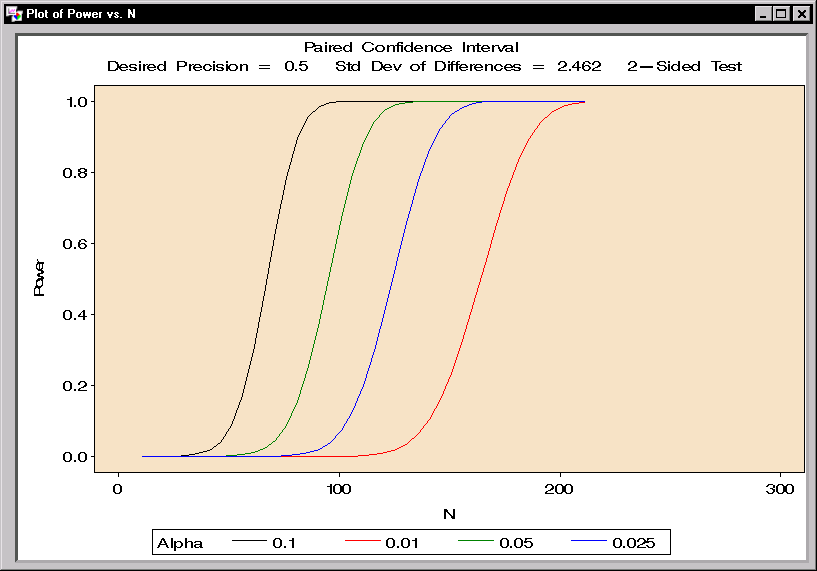Figure 12.9: Plot for Paired Confidence Interval

The requested plot is displayed in Figure 12.9 and includes one power curve for each specified alpha value.

Top of Page