| The QUANTREG Procedure |
| Linear Test |
Two tests are available in the QUANTREG procedure for the linear null hypothesis 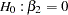 . Here
. Here 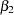 denotes a subset of the parameters, where the parameter vector
denotes a subset of the parameters, where the parameter vector 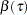 is partitioned as
is partitioned as 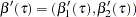 , and the covariance matrix
, and the covariance matrix 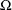 for the parameter estimates is partitioned correspondingly as
for the parameter estimates is partitioned correspondingly as 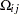 with
with  ; and
; and 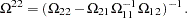
The Wald test statistic, which is based on the estimated coefficients for the unrestricted model, is given by
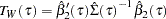 |
where 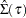 is an estimator of the covariance of
is an estimator of the covariance of 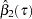 . The QUANTREG procedure provides two estimators for the covariance, as described in the previous section. The estimator based on the asymptotic covariance is
. The QUANTREG procedure provides two estimators for the covariance, as described in the previous section. The estimator based on the asymptotic covariance is
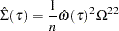 |
where 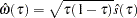 and
and 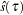 is the estimated sparsity function. The estimator based on the bootstrap covariance is the empirical covariance of the MCMB samples.
is the estimated sparsity function. The estimator based on the bootstrap covariance is the empirical covariance of the MCMB samples.
The likelihood ratio test is based on the difference between the objective function values in the restricted and unrestricted models. Let 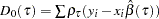 and
and 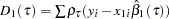 , and set
, and set
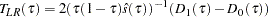 |
where 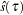 is the estimated sparsity function.
is the estimated sparsity function.
Koenker and Machado (1999) prove that these two tests are asymptotically equivalent and that the distributions of the test statistics converge to 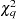 under the null hypothesis, where
under the null hypothesis, where  is the dimension of
is the dimension of 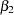 .
.
Copyright © 2009 by SAS Institute Inc., Cary, NC, USA. All rights reserved.