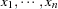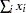| The MCMC Procedure |
| Some Useful SAS Functions |
SAS Function |
Definition |
|---|---|
abs(x) |
|
airy(x) |
returns the value of the AIRY function. |
beta(x1, x2) |
|
call logistic(x) |
|
call softmax(x1,...,xn) |
each element is replaced by |
call stdize(x1,...,xn) |
standardize values |
cdf |
cumulative distribution function |
cdf(’normal’, x, 0, 1) |
standard normal cumulative distribution function |
comb(x1, x2) |
|
constant(’.’) |
calculate commonly used constants |
cos(x) |
cosine(x) |
css(x1, ..., xn) |
|
cv(x1, ..., xn) |
std(x) / mean(x) * 100 |
dairy(x) |
derivative of the AIRY function |
dimN(m) |
returns the numbers of elements in the Nth dim of array |
(x1 eq x2) |
returns 1 if x1 = x2; 0 otherwise |
x1**x2 |
|
geomean(x1, ..., xn) |
|
difN(x) |
returns differences between the argument and its Nth lag |
digamma(x1) |
|
erf(x) |
|
erfc(x) |
1 - erf(x) |
fact(x) |
|
floor(x) |
greatest integer |
gamma(x) |
|
harmean(x1, ..., xn) |
|
ibessel(nu, x, kode) |
modified Bessel function of order nu evaluated at |
jbessel(nu, x) |
Bessel function of order nu evaluated at |
lagN(x) |
returns values from a queue |
largest(k, x1, ..., xn) |
the |
lgamma(x) |
|
lgamma(x+1) |
|
log(x), logN(x) |
|
logbeta(x1, x2) |
lgamma( |
logcdf |
log of a left cumulative distribution function |
logpdf |
log of a probability density (mass) function |
logsdf |
log of a survival function |
max(x1, x2) |
returns |
mean(of x1-xn) |
|
median(of x1-xn) |
returns the median of nonmissing values |
min(x1, x2) |
returns |
missing(x) |
returns 1 if |
mod(x1, x2) |
returns the remainder from |
n(x1, ..., xn) |
returns number of nonmissing values |
nmiss(of y1-yn) |
number of missing values |
quantile |
computes the quantile from a specific distribution |
probability density (mass) functions |
|
perm(n, r) |
|
put |
returns a value that uses a specified format |
round(x) |
rounds x |
rms(of x1-xn) |
|
sdf |
survival function |
sign(x) |
returns |
sin(x) |
sine( |
smallest( |
the |
sortn(of x1-xn) |
sorts the values of the variables |
sqrt(x) |
|
std(x1, ..., xn) |
standard deviation of |
sum(of x:) |
|
trigamma(x) |
derivative of the DIGAMMA( |
uss(of x1-xn) |
uncorrected sum of squares |
Here are examples of some commonly used transformations:
Copyright © 2009 by SAS Institute Inc., Cary, NC, USA. All rights reserved.




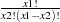

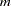
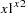


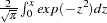
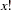
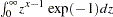


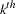 largest element
largest element 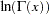

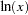
 ) + lgamma(
) + lgamma( ) - lgamma(
) - lgamma( )
)  ;
; 
 ;
; 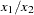
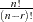

 if
if  ;
; 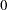 if
if 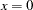 ;
; 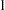 if
if 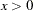
 , x1, ..., en )
, x1, ..., en ) 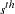 smallest component of
smallest component of