Beta
Previous Page|Next Page

Beta

The density function of the beta distribution is

\[ f(x) = \frac{\Gamma (\alpha + \beta )}{\Gamma (\alpha )\Gamma (\beta )(b - a)^{\alpha + \beta - 1}}(x - a)^{\alpha - 1}(b - x)^{\beta - 1} \]

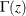for $a \le x \le b$. The gamma function $\Gamma (z)$ is defined for any real number $z > 0$ as

\[ \Gamma (z) = \int _{0}^{\infty }t^{z - 1}e^{-t}dt \]

Parameters:

$a$

is the minimum value, 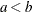$a < b$.

$b$

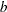
is the maximum value.

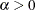
$\alpha > 0$

is a shape parameter.

$\beta > 0$

is a shape parameter.

Previous Page|Next Page|Top of Page
Copyright © SAS Institute Inc. All Rights Reserved.