The FACTEX Procedure
Block Factors
If your design involves blocks, additional confounding criteria need to be considered. Blocks are introduced into designs
by means of block pseudo-factors. (See Types of Factors for details.) A design for q-level factors in 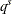 blocks contains s block pseudo-factors. Denoting the levels of these factors for any given run by
blocks contains s block pseudo-factors. Denoting the levels of these factors for any given run by  , the index of the block in which the run occurs is given by
, the index of the block in which the run occurs is given by
![\[ B_1 + qB_2 + q^2B_3 + \ldots + q^{s-1}B_ s \]](images/qcug_factex0116.png)
For each block to occur in the design, every possible combination of block pseudo-factors must occur. This can happen only if all main effects and interactions between the block factors are estimable, which leads to yet another criterion for the confounding rules. Moreover, the effects you want to estimate cannot be confounded with blocks. In general:
-
no generalized block pseudo-factors can be confounded with zero
-
no generalized interactions between block pseudo-factors and effects you want to estimate can be confounded with zero