The FACTEX procedure constructs a fractional design for 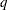-level factors by using the Galois field (or finite field) of size 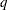. This is a system with 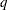elements and two operations 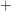and , which satisfy the usual mathematical axioms for addition and multiplication. When 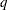is a prime number, finite field arithmetic is equivalent to regular integer arithmetic modulo 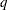. When 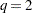, addition of the two elements of the finite field is equivalent to multiplication of the integers 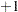and . Since designs for factors with levels 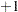and are the factorial designs most commonly covered in textbooks, the arithmetic for fractional factorial designs is usually shown in multiplicative form. However, throughout this section a more general notation is used.

A design for 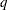-level factors in runs constructed by the FACTEX procedure has the following general form. The first factors are taken to index the runs in the design, with one run for each different combination of the levels of these factors, where the levels run from 0 to . These factors are called run-indexing factors. For a particular run, the value of any other factor in the design is derived from the levels 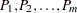of the run-indexing factors by means of confounding rules. These rules are of the general form

where all the arithmetic is performed in the finite field of size 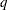. The linear combination on the right-hand side of the preceding equation is called a generalized interaction between the run-indexing factors. A generalized interaction is part of the statistical interaction between the factors with nonzero coefficients in the linear combination. The factor is said to be confounded or aliased with this generalized interaction; two terms are confounded when the levels they take in the design yield identical partitions of the runs, so that their effects cannot be distinguished. The confounding rules characterize the design, and the problem of constructing the design reduces to finding suitable confounding rules.