Multivariate Analysis: Correlation Analysis
Variables Tab
You can use the Variables tab to specify the numerical variables for the analysis. The Variables tab is shown in Figure 25.2.
The variables in the list correspond to variables in the VAR statement of the CORR procedure. The variables in the list correspond to variables in the WITH statement of the CORR procedure.
The simplest way to analyze correlations is to add the variables of interest to the list, as in the example earlier in this chapter.
If the list is empty, the correlation matrix is symmetric. If you request a matrix of pairwise scatter plots (on the Plots tab), you will get plots for pairs of variables in the lower triangular portion of the matrix.
If the list is not empty, the correlation matrix is not symmetric. If you specify 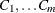 as the Y variables and
as the Y variables and 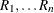 as the WITH variables,
then the (i, j) cell of the correlation matrix will be the correlation of
as the WITH variables,
then the (i, j) cell of the correlation matrix will be the correlation of 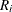 with
with 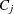 . If you request a matrix of pairwise scatter plots, you will get
. If you request a matrix of pairwise scatter plots, you will get 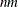 plots, arranged in n rows and m columns.
plots, arranged in n rows and m columns.
The list is rarely used. The variables in this list correspond to variables in the PARTIAL statement of the CORR procedure. A partial correlation measures the strength of a relationship between two variables, while controlling the effect of other variables. The Pearson partial correlation between two variables, after controlling for variables in the PARTIAL statement, is equivalent to the Pearson correlation between the residuals of the two variables after regression on the controlling variables.
If there are variables in the list, then the following conditions hold:
-
You cannot request Hoeffding’s D correlation statistic.
-
Observations with missing values are excluded from the analysis.