The MODEL Procedure
The options ITPRINT, ITDETAILS, XPX, I, and ITALL specify a detailed listing of each iteration of the minimization process.
The ITPRINT information is selected whenever any iteration information is requested.
The following information is displayed for each iteration:
- N
-
is the number of usable observations.
- Objective
-
is the corrected objective function value.
- Trace(S)
-
is the trace of the S matrix.
- subit
-
is the number of subiterations required to find a
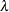 or a damping factor that reduces the objective function.
or a damping factor that reduces the objective function.
- R
-
is the R convergence measure.
The estimates for the parameters at each iteration are also printed.
The additional values printed for the ITDETAILS option are:
- Theta
-
is the angle in degrees between
 , the parameter change vector, and the negative gradient of the objective function.
, the parameter change vector, and the negative gradient of the objective function.
- Phi
-
is the directional derivative of the objective function in the
 direction scaled by the objective function.
direction scaled by the objective function.
- Stepsize
-
is the value of the damping factor used to reduce
 if the Gauss-Newton method is used.
if the Gauss-Newton method is used.
- Lambda
-
is the value of
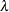 if the Marquardt method is used.
if the Marquardt method is used.
- Rank(XPX)
-
is the rank of the
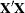 matrix (output if the projected Jacobian crossproducts matrix is singular).
matrix (output if the projected Jacobian crossproducts matrix is singular).
The definitions of PPC and R are explained in the section Convergence Criteria. When the values of PPC are large, the parameter associated with the criteria is displayed in parentheses after the value.
The XPX and the I options select the printing of the augmented ![]() matrix and the augmented
matrix and the augmented ![]() matrix after a sweep operation (Goodnight, 1979) has been performed on it. An example of the output from the following statements is shown in Figure 19.36.
matrix after a sweep operation (Goodnight, 1979) has been performed on it. An example of the output from the following statements is shown in Figure 19.36.
proc model data=test2; y1 = a1 * x2 * x2 - exp( d1*x1); y2 = a2 * x1 * x1 + b2 * exp( d2*x2); fit y1 y2 / itall XPX I ; run;
Figure 19.36: XPX and I Options Output
| Cross Products for System At OLS Iteration 0 | ||||||
|---|---|---|---|---|---|---|
| a1 | d1 | a2 | b2 | d2 | Residual | |
| a1 | 1839468 | -33818.35 | 0.0 | 0.00 | 0.000000 | 3879959 |
| d1 | -33818 | 1276.45 | 0.0 | 0.00 | 0.000000 | -76928 |
| a2 | 0 | 0.00 | 42925.0 | 1275.15 | 0.154739 | 470686 |
| b2 | 0 | 0.00 | 1275.2 | 50.01 | 0.003867 | 16055 |
| d2 | 0 | 0.00 | 0.2 | 0.00 | 0.000064 | 2 |
| Residual | 3879959 | -76928.14 | 470686.3 | 16055.07 | 2.329718 | 24576144 |
| XPX Inverse for System At OLS Iteration 0 | ||||||
|---|---|---|---|---|---|---|
| a1 | d1 | a2 | b2 | d2 | Residual | |
| a1 | 0.000001 | 0.000028 | 0.000000 | 0.0000 | 0.00 | 2 |
| d1 | 0.000028 | 0.001527 | 0.000000 | 0.0000 | 0.00 | -9 |
| a2 | 0.000000 | 0.000000 | 0.000097 | -0.0025 | -0.08 | 6 |
| b2 | 0.000000 | 0.000000 | -0.002455 | 0.0825 | 0.95 | 172 |
| d2 | 0.000000 | 0.000000 | -0.084915 | 0.9476 | 15746.71 | 11931 |
| Residual | 1.952150 | -8.546875 | 5.823969 | 171.6234 | 11930.89 | 10819902 |
The first matrix, labeled "Cross Products," for OLS estimation is
The column labeled Residual in the output is the vector ![]() , which is the gradient of the objective function. The diagonal scalar value
, which is the gradient of the objective function. The diagonal scalar value ![]() is the objective function uncorrected for degrees of freedom. The second matrix, labeled "XPX Inverse," is created through
a sweep operation on the augmented
is the objective function uncorrected for degrees of freedom. The second matrix, labeled "XPX Inverse," is created through
a sweep operation on the augmented ![]() matrix to get:
matrix to get:
Note that the residual column is the change vector used to update the parameter estimates at each iteration. The corner scalar element is used to compute the R convergence criteria.
The ITALL option, in addition to causing the output of all of the preceding options, outputs the S matrix, the inverse of the S matrix, the CROSS matrix, and the swept CROSS matrix. An example of a portion of the CROSS matrix for the preceding example is shown in Figure 19.37.
Figure 19.37: ITALL Option Crossproducts Matrix Output
| Crossproducts Matrix At OLS Iteration 0 | ||||||||
|---|---|---|---|---|---|---|---|---|
| 1 | @PRED.y1/@a1 | @PRED.y1/@d1 | @PRED.y2/@a2 | @PRED.y2/@b2 | @PRED.y2/@d2 | RESID.y1 | RESID.y2 | |
| 1 | 50.00 | 6409 | -239.16 | 1275.0 | 50.00 | 0.003803 | 14700 | 16053 |
| @PRED.y1/@a1 | 6409.08 | 1839468 | -33818.35 | 187766.1 | 6409.88 | 0.813934 | 3879959 | 4065028 |
| @PRED.y1/@d1 | -239.16 | -33818 | 1276.45 | -7253.0 | -239.19 | -0.026177 | -76928 | -85084 |
| @PRED.y2/@a2 | 1275.00 | 187766 | -7253.00 | 42925.0 | 1275.15 | 0.154739 | 420583 | 470686 |
| @PRED.y2/@b2 | 50.00 | 6410 | -239.19 | 1275.2 | 50.01 | 0.003867 | 14702 | 16055 |
| @PRED.y2/@d2 | 0.00 | 1 | -0.03 | 0.2 | 0.00 | 0.000064 | 2 | 2 |
| RESID.y1 | 14699.97 | 3879959 | -76928.14 | 420582.9 | 14701.77 | 1.820356 | 11827102 | 12234106 |
| RESID.y2 | 16052.76 | 4065028 | -85083.68 | 470686.3 | 16055.07 | 2.329718 | 12234106 | 12749042 |